Advertisements
Advertisements
Question
In a shopping mall there is a hall of cuboid shape with dimension 800 × 800 × 720 units, which needs to be added the facility of an escalator in the path as shown by the dotted line in the figure. Find the minimum total length of the escalator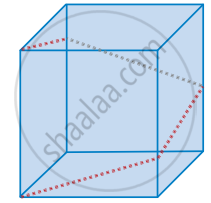
Solution
Give the dimension of the cube = 800 × 800 × 720
Minimum total length of the escalator:
Shape of the hall is cuboid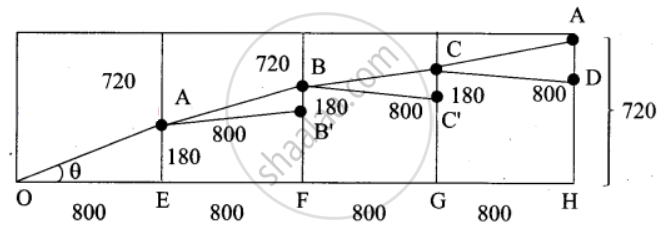
The path of the escalator is
From OA to AB to BC to CD
OE = 800
EA = `1/4 xx "Height of the building"`
EA = `1/4 xx 720` = 180
Since there are four steps for the escalator
∴ OA2 = OE2 + EA2
= 8002 + 1802
= (40 × 20)2 + (9 × 20)2
= 402 × 202 + 92 × 202
= 202 (402 + 92)
= 202 (1600 + 81)
= 202 × 1681
OA2 = 202 × 412
OA = `sqrt(20^2 xx 41^2)`
= 20 × 41
= 820
Since ∆OAE ≡ ∆ABB’ ≡ ∆ BCC ≡ ∆CDD’
We have OA = AB = BC = CD
Total length of the escalator
= OA + AB + BC + CD
= 820 + 820 + 820 + 820
= 4 × 820
= 3280
Minimum length of the escalator = 3280 units
APPEARS IN
RELATED QUESTIONS
Find the slope of the following line which passes through the points:
C(−2, 3), D(5, 7)
Find the slope of the following line which passes through the points:
E(2, 3), F(2, −1)
A line makes intercepts 3 and 3 on the co-ordinate axes. Find the inclination of the line.
Without using Pythagoras theorem show that points A(4, 4), B(3, 5) and C(−1, −1) are the vertices of a right angled triangle.
Answer the following question:
Find the value of k if the slope of the line passing through the points P(3, 4), Q(5, k) is 9
Find the equation of the lines passing through the point (1,1) and (– 2, 3)
An object was launched from a place P in constant speed to hit a target. At the 15th second, it was 1400 m from the target, and at the 18th second 800 m away. Find time taken to hit the target
Find the equation of the line, if the perpendicular drawn from the origin makes an angle 30° with x-axis and its length is 12
Find the equation of the straight lines passing through (8, 3) and having intercepts whose sum is 1
Show that the points (1, 3), (2, 1) and `(1/2, 4)` are collinear, by using concept of slope
Show that the points (1, 3), (2, 1) and `(1/2, 4)` are collinear, by using any other method
A spring was hung from a hook in the ceiling. A number of different weights were attached to the spring to make it stretch, and the total length of the spring was measured each time is shown in the following table
| Weight (kg) | 2 | 4 | 5 | 8 |
| Length (cm) | 3 | 4 | 4.5 | 6 |
Find the equation relating the length of the spring to the weight on it
A spring was hung from a hook in the ceiling. A number of different weights were attached to the spring to make it stretch, and the total length of the spring was measured each time is shown in the following table
| Weight (kg) | 2 | 4 | 5 | 8 |
| Length (cm) | 3 | 4 | 4.5 | 6 |
What is the actual length of the spring
A spring was hung from a hook in the ceiling. A number of different weights were attached to the spring to make it stretch, and the total length of the spring was measured each time is shown in the following table
| Weight (kg) | 2 | 4 | 5 | 8 |
| Length (cm) | 3 | 4 | 4.5 | 6 |
How long will the spring be when 6 kilograms of weight on it?
A point on the straight line, 3x + 5y = 15 which is equidistant from the coordinate, axes will lie only in ______.
The number of possible tangents which can be drawn to the curve 4x2 – 9y2 = 36, which are perpendicular to the straight line 5x + 2y – 10 = 0 is ______.
Find the coordinates of the point which divides the line segment joining the points (1, –2, 3) and (3, 4, –5) internally in the ratio 2 : 3.
Find the transformed equation of the straight line 2x – 3y + 5 = 0, when the origin is shifted to the point (3, –1) after translation of axes.
