Question

In a ΔABC, AD is the bisector of ∠A, meeting side BC at D.

If BD = 2.5cm, AB = 5cm and AC = 4.2cm, find DC.

Answer 1

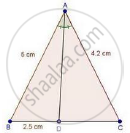
We have,

∠BAD = ∠CAD

We know that, the internal bisector of an angle of a triangle divides the opposite side internally in the ratio of the sides containing the angle.

`therefore"BD"/"DC"="AB"/"AC"`

`rArr2.5/"DC"=5/4.2`

`rArr"DC"=(2.5xx4.2)/5`

`=(25xx42)/(5xx100)=(5xx42)/100=210/100=2.1 `cm

∴ DC = 2.1 cm