Advertisements
Advertisements
Question
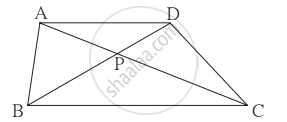
In ▢ABCD, seg AD || seg BC. Diagonal AC and diagonal BD intersect each other in point P. Then show that `"AP"/"PD" = "PC"/"BP"`.
Solution
Given: ▢ABCD is a parallelogram. seg AD || seg BC and BD is their transversal.
To prove: `"AP"/"PD" = "PC"/"BP"`
Proof:
seg AD || seg BC and BD is their transversal. ...(Given)
∴ ∠DBC ≅ ∠BDA ...(Alternate angles)
∴ ∠PBC ≅ ∠PDA ...(i) [D−P−B]
In △PBC and △PDA,
∠PBC ≅ ∠PDA ...[From (i)]
∠BPC ≅ ∠DPA ...(Verticall opposite angles)
By AA test of similarity,
∴ △APD ∼ CPB
∴ `"AP"/"PC" = "PD"/"PB"` ...(Corresponding sides of similar triangles)
∴ `"AP"/"PD" = "PC"/"PB"` ...(By alternendo)
Hence proved.
APPEARS IN
RELATED QUESTIONS
Given below is the triangle and length of line segments. Identify in the given figure, ray PM is the bisector of ∠QPR.

Measures of some angles in the figure are given. Prove that `"AP"/"PB" = "AQ"/"QC"`.
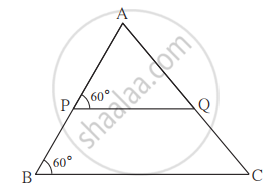
In the given figure, if AB || CD || FE then find x and AE.

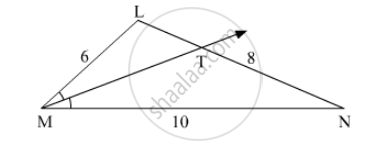
In ∆LMN, ray MT bisects ∠LMN If LM = 6, MN = 10, TN = 8, then Find LT.
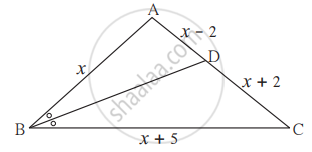
In ∆ABC, seg BD bisects ∠ABC. If AB = x, BC = x + 5, AD = x – 2, DC = x + 2, then find the value of x.
In the given fig, bisectors of ∠B and ∠C of ∆ABC intersect each other in point X. Line AX intersects side BC in point Y. AB = 5, AC = 4, BC = 6 then find `"AX"/"XY"`.

In Δ ABC and Δ PQR,
∠ ABC ≅ ∠ PQR, seg BD and
seg QS are angle bisector.
`If (l(AD))/(l(PS)) = (l(DC))/(l(SR))`
Prove that : Δ ABC ∼ Δ PQR


In ΔABC, ray BD bisects ∠ABC.
If A – D – C, A – E – B and seg ED || side BC, then prove that:
`("AB")/("BC") = ("AE")/("EB")`
Proof :
In ΔABC, ray BD bisects ∠ABC.
∴ `("AB")/("BC") = (......)/(......)` ......(i) (By angle bisector theorem)
In ΔABC, seg DE || side BC
∴ `("AE")/("EB") = ("AD")/("DC")` ....(ii) `square`
∴ `("AB")/square = square/("EB")` [from (i) and (ii)]

In ΔABC, ∠ACB = 90°. seg CD ⊥ side AB and seg CE is angle bisector of ∠ACB.
Prove that: `(AD)/(BD) = (AE^2)/(BE^2)`.
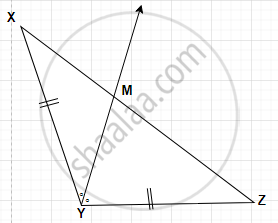
In the figure, ray YM is the bisector of ∠XYZ, where seg XY ≅ seg YZ, find the relation between XM and MZ.
Draw seg AB = 6.8 cm and draw perpendicular bisector of it.
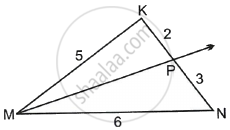
From the information given in the figure, determine whether MP is the bisector of ∠KMN.
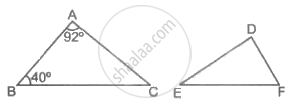
If ΔABC ∼ ΔDEF such that ∠A = 92° and ∠B = 40°, then ∠F = ?
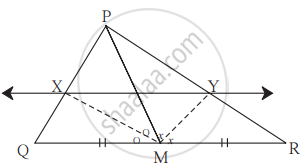
In ∆PQR seg PM is a median. Angle bisectors of ∠PMQ and ∠PMR intersect side PQ and side PR in points X and Y respectively. Prove that XY || QR.
Complete the proof by filling in the boxes.
solution:
In ∆PMQ,
Ray MX is the bisector of ∠PMQ.
∴ `("MP")/("MQ") = square/square` .............(I) [Theorem of angle bisector]
Similarly, in ∆PMR, Ray MY is the bisector of ∠PMR.
∴ `("MP")/("MR") = square/square` .............(II) [Theorem of angle bisector]
But `("MP")/("MQ") = ("MP")/("MR")` .............(III) [As M is the midpoint of QR.]
Hence MQ = MR
∴ `("PX")/square = square/("YR")` .............[From (I), (II) and (III)]
∴ XY || QR .............[Converse of basic proportionality theorem]
