Advertisements
Advertisements
Question
In covering a distance s metres, a circular wheel of radius r metres makes `s/(2πr)` revolutions. Is this statement true? Why?
Solution
The distance covered in one revolution is 2πr, So, number of revolutions made in covering a distance of s m = `s/(2πr)`.
APPEARS IN
RELATED QUESTIONS
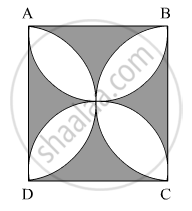
In Fig. 4, ABCD is a square of side 14 cm. Semi-circles are drawn with each side of square as diameter. Find the area of the shaded region.
(use `pi=22/7`)
The length of minute hand of a clock is 14 cm. Find the area swept by the minute hand in one minute. (Use π = 22/7)
Find the circumferences of a circle whose radius is 7 cm.
The circumference of a circle is 22 cm. Find the area of its quadrant.
What is the perimeter of a square which circumscribes a circle of radius a cm?
The circumference of a circle is 8 cm. Find the area of the sector whose central angle is 72°.
A bucket is raised from a well by means of a rope wound round a wheel of diameter 35 cm. If the bucket ascends in 2 minutes with a uniform speed of 1.1 m per sec, calculate the number of complete revolutions the wheel makes in raising the bucket.
Find the diameter of the sphere for the following :
Surface Area = 221. 76 cm2
Find the dimensions of a rectangular park whose perimeter is 60 m and area 200 m2.
Areas of two circles are equal. Is it necessary that their circumferences are equal? Why?
