Question

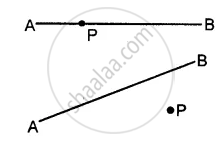
In each of the following, draw perpendicular through point P to the line segment AB :

(i)

(ii)

(iii)

Answer 1

(i) Steps of Construction :

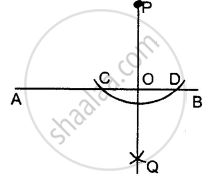
1. With P as a centre, draw an arc of a suitable radius which cuts AB at points C and D.
2. With C and D as centres, draw arcs of equal radii and let these arcs intersect each other at point Q.
   [The radius of these arcs must be more than half of CD and both the arcs must be drawn on the other side]
3. Join P and Q
4. Let PQ cut AB at the point O.
   Thus, OP is the required perpendicular clearly, ∠AOP = ∠BOP = 90°

(ii) Steps of Construction :

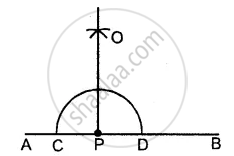
1. With P as a centre, draw an arc of any suitable radius which cuts AB at points C and D.
2. With C and D as centres, draw arcs of equal radii. Which intersect each other at point A.
   [This radius must be more than half of CD and let these arc intersect each other at the point 0]
3. Join P and O. Then OP is the required perpendicular.
   ∠OPA = ∠OPB = 90°

(iii) Steps of Construction :

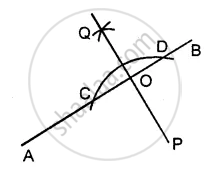
1. With P as a centre, draw an arc of any suitable radius which cuts AB at points C and D.
2. With C and D as a centre, draw arcs of equal radii
   [The radius of these arcs must be more than half of CD and both the arcs must be drawn on the other side.]
   and let these arcs intersect each other at the point Q.
3. Join Q and P. Let QP cut AB at the point O. Then OP is the required perpendicular.
   Clearly, ∠AOP = ∠BOP = 90°