Advertisements
Advertisements
Question
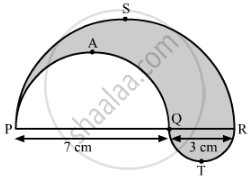
In PSR, RTQ and PAQ are three semicircles of diameters 10 cm, 3 cm and 7 cm respectively. Find the perimeter of the shaded region. [Use π=3.14]
Solution
Radius of semicircle PSR = 1/2×10 cm= 5 cm
Radius of semicircle RTQ = 1/2×3 cm = 1.5 cm
Radius of semicircle PAQ = 1/2×7 cm = 3.5 cm
Perimeter of the shaded region = Circumference of semicircle PSR + Circumference of semicircle RTQ + Circumference of semicircle PAQ
`= [1/2xx 2π(5) + 1/2xx 2π(1.5) + 1/2xx 2π(3.5)] cm`
`= π (5 + 1.5 + 3.5) cm`
`= 3.14 xx 10 cm`
`= 31.4 cm`
APPEARS IN
RELATED QUESTIONS
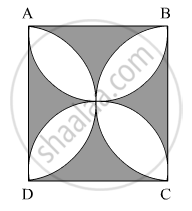
In Fig. 4, ABCD is a square of side 14 cm. Semi-circles are drawn with each side of square as diameter. Find the area of the shaded region.
(use `pi=22/7`)
An arc of a circle is of length 5π cm and the sector it bounds has an area of 20 π cm2. Find the radius of the circle.
The radii of two circles are 19 cm and 9 cm respectively. Find the radius of the circle which has circumference equal to the sum of the circumferences of the two circles.
Find the circumference and area of circle of radius 4.2 cm
An arc of length 20𝜋 cm subtends an angle of 144° at centre of circle. Find the radius of the circle.
The side of a square is 10 cm. Find the area of the inscribed circle [π = 3.14]
Find the area and perimeter of the following semi-circle :
Diameter= 7 cm
Find the area and perimeter of the following semi-circle :
Diameter= 5.6 cm
Find the radius and area of the circle which has circumference equal to the sum of circumferences of the two circles of radii 3 cm and 4 cm respectively.
The area between the circumference of two concentric circles is 2464cm2. If the inner circle has a circumference of 132cm, calculate the radius of the outer circle.
