Question

In the following figure, ABCD is a rhombus and DCFE is a square.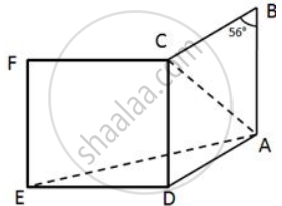

If ∠ABC =56°, find:
(i) ∠DAE
(ii) ∠FEA
(iii) ∠EAC
(iv) ∠AEC

Answer 1

ABCD is a rhombus.
⇒ AD = CD and ∠ADC = ∠ABC = 56°
DCFE is a square.
⇒ ED = CD and ∠FED = ∠EDC = ∠DCF = ∠CFE = 90°
⇒ AD = CD = ED
In ΔADE,
AD = ED
⇒ ∠DAE = ∠AED                ...(i)
∠DAE + ∠AED + ∠ADE = 180°
⇒ 2∠DAE + 146° = 180°            ....( Since ∠ADE = ∠EDC + ∠ADC = 90° + 56° = 146° )
⇒ 2∠DAE = 34°
⇒ ∠DAE = 17°
⇒ ∠DEA = 17°                   ....(ii)

In ABCD,
∠ABC + ∠BCD + ∠ADC + ∠DAB = 360°
⇒ 56° + 56° + 2 ∠DAB = 360°   ....( ∵ Opposite angles of a rhombus are equal.)
⇒ 2∠DAB = 248°
⇒ ∠DAB = 124°
We know that diagonals of a rhombus, bisect its angles.

⇒ ∠DAC = `(124°)/2` = 62°

⇒ ∠EAC = ∠DAC - ∠DAE = 62° - 17° = 45°
Now,
∠FEA = ∠FED - ∠DEA  
          = 90° - 17°             ....( From(ii) and each angle of a square is 90° )
        = 73°       
We know that diagonals of a square bisect its angles.
⇒ ∠CED = `(90°)/2` = 45°
So,
∠AEC = ∠CED - ∠DEA
          = 45° - 17°
          = 28°
Hence, ∠DAE = 17°, ∠FEA = 73°, ∠EAC = 45° and ∠AEC = 28°.