Advertisements
Chapters
2: Compound Interest (Without using formula)
3: Compound Interest (Using Formula)
4: Expansions (Including Substitution)
5: Factorisation
6: Simultaneous (Linear) Equations (Including Problems)
7: Indices (Exponents)
8: Logarithms
9: Triangles [Congruency in Triangles]
10: Isosceles Triangles
11: Inequalities
12: Mid-point and Its Converse [ Including Intercept Theorem]
13: Pythagoras Theorem [Proof and Simple Applications with Converse]
▶ 14: Rectilinear Figures [Quadrilaterals: Parallelogram, Rectangle, Rhombus, Square and Trapezium]
15: Construction of Polygons (Using ruler and compass only)
16: Area Theorems [Proof and Use]
17: Circle
18: Statistics
19: Mean and Median (For Ungrouped Data Only)
20: Area and Perimeter of Plane Figures
21: Solids [Surface Area and Volume of 3-D Solids]
22: Trigonometrical Ratios [Sine, Consine, Tangent of an Angle and their Reciprocals]
23: Trigonometrical Ratios of Standard Angles [Including Evaluation of an Expression Involving Trigonometric Ratios]
24: Solution of Right Triangles [Simple 2-D Problems Involving One Right-angled Triangle]
25: Complementary Angles
26: Co-ordinate Geometry
27: Graphical Solution (Solution of Simultaneous Linear Equations, Graphically)
28: Distance Formula
![Selina solutions for Concise Mathematics [English] Class 9 ICSE chapter 14 - Rectilinear Figures [Quadrilaterals: Parallelogram, Rectangle, Rhombus, Square and Trapezium] Selina solutions for Concise Mathematics [English] Class 9 ICSE chapter 14 - Rectilinear Figures [Quadrilaterals: Parallelogram, Rectangle, Rhombus, Square and Trapezium] - Shaalaa.com](/images/concise-mathematics-english-class-9-icse_6:b313c06da7fb4b0f885a06c3b5e4e4fa.jpg)
Advertisements
Solutions for Chapter 14: Rectilinear Figures [Quadrilaterals: Parallelogram, Rectangle, Rhombus, Square and Trapezium]
Below listed, you can find solutions for Chapter 14 of CISCE Selina for Concise Mathematics [English] Class 9 ICSE.
Selina solutions for Concise Mathematics [English] Class 9 ICSE 14 Rectilinear Figures [Quadrilaterals: Parallelogram, Rectangle, Rhombus, Square and Trapezium] Exercise 14 (A) [Pages 168 - 169]
The sum of the interior angles of a polygon is four times the sum of its exterior angles.
Find the number of sides in the polygon.
The angles of a pentagon are in the ratio 4: 8: 6: 4: 5.
Find each angle of the pentagon.
One angle of a six-sided polygon is 140o and the other angles are equal.
Find the measure of each equal angle.
In a polygon, there are 5 right angles and the remaining angles are equal to 195o each. Find the number of sides in the polygon.
Three angles of a seven-sided polygon are 132o each and the remaining four angles are equal. Find the value of each equal angle.
Two angles of an eight-sided polygon are 142o and 176o. If the remaining angles are equal to each other; find the magnitude of each of the equal angles.
In a pentagon ABCDE, AB is parallel to DC and ∠A: ∠E : ∠D = 3: 4: 5. Find angle E.
AB, BC, and CD are the three consecutive sides of a regular polygon. If BAC = 15°;
Find:
- Each interior angle of the polygon.
- Each exterior angle of the polygon.
- The number of sides of the polygon.
The ratio between an exterior angle and an interior angle of a regular polygon is 2 : 3. Find the number of sides in the polygon.
The difference between an exterior angle of (n - 1) sided regular polygon and an exterior angle of (n + 2) sided regular polygon is 6° find the value of n.
Two alternate sides of a regular polygon, when produced, meet at the right angle.
Find:
(i)The value of each exterior angle of the polygon;
(ii) The number of sides in the polygon.
Selina solutions for Concise Mathematics [English] Class 9 ICSE 14 Rectilinear Figures [Quadrilaterals: Parallelogram, Rectangle, Rhombus, Square and Trapezium] Exercise 14 (B) [Pages 175 - 176]
State, 'true' or 'false'
The diagonals of a rectangle bisect each other.
True
False
State, 'true' or 'false'
The diagonals of a quadrilateral bisect each other.
True
False
State, 'true' or 'false'
The diagonals of a parallelogram bisect each other at right angle.
True
False
State, 'true' or 'false'
Each diagonal of a rhombus bisects it.
True
False
State, 'true' or 'false'
The quadrilateral, whose four sides are equal, is a square.
True
False
State, 'true' or 'false'
Every rhombus is a parallelogram.
True
False
State, 'true' or 'false'
Every parallelogram is a rhombus.
True
False
State, 'true' or 'false'
Diagonals of a rhombus are equal.
True
False
State, 'true' or 'false'
If two adjacent sides of a parallelogram are equal, it is a rhombus.
True
False
State, 'true' or 'false'
If the diagonals of a quadrilateral bisect each other at right angle, the quadrilateral is a square.
True
False
In the figure, given below, AM bisects angle A and DM bisects angle D of parallelogram ABCD. Prove that: ∠AMD = 90°.

In the following figure, AE and BC are equal and parallel and the three sides AB, CD, and DE are equal to one another. If angle A is 102o. Find angles AEC and BCD.
In a square ABCD, diagonals meet at O. P is a point on BC such that OB = BP.
Show that:
- ∠POC = `[ 22 ( 1°)/( 2 ) ]`
- ∠BDC = 2 ∠POC
- ∠BOP = 3 ∠CPO
The given figure shows a square ABCD and an equilateral triangle ABP. 
Calculate: (i) ∠AOB
(ii) ∠BPC
(iii) ∠PCD
(iv) Reflex ∠APC
In the given figure ABCD is a rhombus with angle A = 67°

If DEC is an equilateral triangle, calculate:
- ∠CBE
- ∠DBE
In the following figures, ABCD is a parallelogram.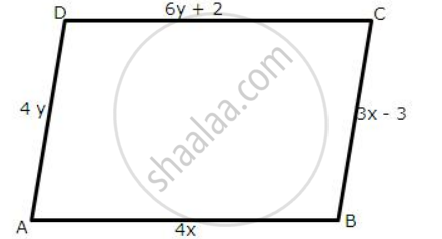
find the values of x and y.
In the following figures, ABCD is a parallelogram.

Find the values of x and y.
The angles of a quadrilateral are in the ratio 3: 4: 5: 6. Show that the quadrilateral is a trapezium.
In a parallelogram ABCD, AB = 20 cm and AD = 12 cm. The bisector of angle A meets DC at E and BC produced at F.
Find the length of CF.
In parallelogram ABCD, AP and AQ are perpendiculars from the vertex of obtuse angle A as shown.
If ∠x: ∠y = 2: 1.
find angles of the parallelogram.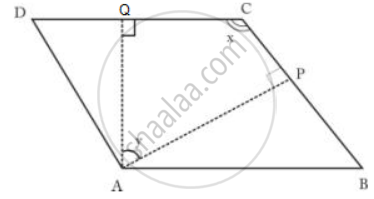
Selina solutions for Concise Mathematics [English] Class 9 ICSE 14 Rectilinear Figures [Quadrilaterals: Parallelogram, Rectangle, Rhombus, Square and Trapezium] Exercise 14 (C) [Pages 181 - 183]
E is the mid-point of side AB and F is the mid-point of side DC of parallelogram ABCD. Prove that AEFD is a parallelogram.
The diagonal BD of a parallelogram ABCD bisects angles B and D. Prove that ABCD is a rhombus.
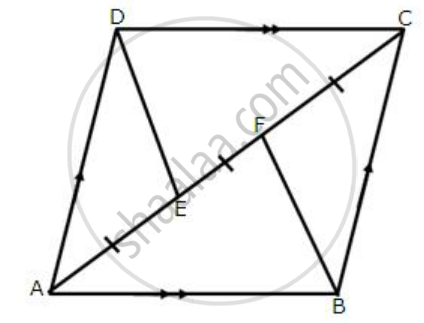
The alongside figure shows a parallelogram ABCD in which AE = EF = FC.
Prove that:
- DE is parallel to FB
- DE = FB
- DEBF is a parallelogram.
In the alongside diagram, ABCD is a parallelogram in which AP bisects angle A and BQ bisects angle B.

Prove that:
- AQ = BP
- PQ = CD
- ABPQ is a parallelogram.
In the given figure, ABCD is a parallelogram.
Prove that: AB = 2 BC.
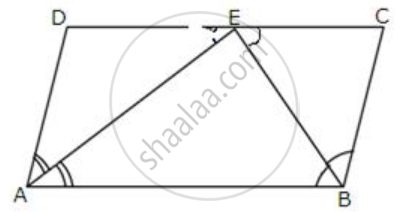
Prove that the bisectors of opposite angles of a parallelogram are parallel.
Prove that the bisectors of interior angles of a parallelogram form a rectangle.
Prove that the bisectors of the interior angles of a rectangle form a square.
In parallelogram ABCD, the bisector of angle A meets DC at P and AB = 2 AD.
Prove that:
(i) BP bisects angle B.
(ii) Angle APB = 90o.
Points M and N are taken on the diagonal AC of a parallelogram ABCD such that AM = CN. Prove that BMDN is a parallelogram.
In the following figure, ABCD is a parallelogram. 
Prove that:
(i) AP bisects angle A.
(ii) BP bisects angle B
(iii) ∠DAP + ∠BCP = ∠APB
ABCD is a square. A is joined to a point P on BC and D is joined to a point Q on AB. If AP = DQ;
prove that AP and DQ are perpendicular to each other.
In a quadrilateral ABCD, AB = AD and CB = CD.
Prove that:
- AC bisects angle BAD.
- AC is the perpendicular bisector of BD.
The following figure shows a trapezium ABCD in which AB is parallel to DC and AD = BC. 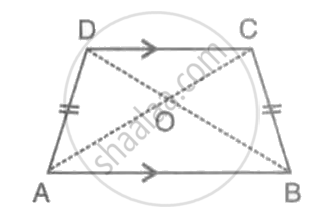
Prove that:
(i) ∠DAB = ∠CBA
(ii) ∠ADC = ∠BCD
(iii) AC = BD
(iv) OA = OB and OC = OD.
In the given figure, AP is the bisector of ∠A and CQ is the bisector of ∠C of parallelogram ABCD. 
Prove that APCQ is a parallelogram.
In case of a parallelogram
prove that:
(i) The bisectors of any two adjacent angles intersect at 90o.
(ii) The bisectors of the opposite angles are parallel to each other.
The diagonals of a rectangle intersect each other at right angles. Prove that the rectangle is a square.
In the following figure, ABCD and PQRS are two parallelograms such that ∠D = 120° and ∠Q = 70°.
Find the value of x.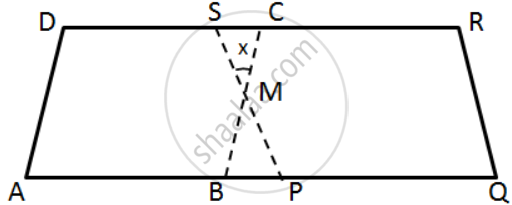
In the following figure, ABCD is a rhombus and DCFE is a square.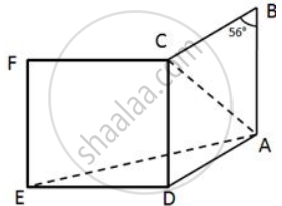
If ∠ABC =56°, find:
(i) ∠DAE
(ii) ∠FEA
(iii) ∠EAC
(iv) ∠AEC
Solutions for 14: Rectilinear Figures [Quadrilaterals: Parallelogram, Rectangle, Rhombus, Square and Trapezium]
![Selina solutions for Concise Mathematics [English] Class 9 ICSE chapter 14 - Rectilinear Figures [Quadrilaterals: Parallelogram, Rectangle, Rhombus, Square and Trapezium] Selina solutions for Concise Mathematics [English] Class 9 ICSE chapter 14 - Rectilinear Figures [Quadrilaterals: Parallelogram, Rectangle, Rhombus, Square and Trapezium] - Shaalaa.com](/images/concise-mathematics-english-class-9-icse_6:b313c06da7fb4b0f885a06c3b5e4e4fa.jpg)
Selina solutions for Concise Mathematics [English] Class 9 ICSE chapter 14 - Rectilinear Figures [Quadrilaterals: Parallelogram, Rectangle, Rhombus, Square and Trapezium]
Shaalaa.com has the CISCE Mathematics Concise Mathematics [English] Class 9 ICSE CISCE solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. Selina solutions for Mathematics Concise Mathematics [English] Class 9 ICSE CISCE 14 (Rectilinear Figures [Quadrilaterals: Parallelogram, Rectangle, Rhombus, Square and Trapezium]) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. Selina textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Concise Mathematics [English] Class 9 ICSE chapter 14 Rectilinear Figures [Quadrilaterals: Parallelogram, Rectangle, Rhombus, Square and Trapezium] are Introduction of Rectilinear Figures, Names of Polygons, Concept of Quadrilaterals, Diagonal Properties of Different Kinds of Parallelograms, Property: The Diagonals of a Rectangle Are of Equal Length., Property: The diagonals of a square are perpendicular bisectors of each other., Types of Quadrilaterals, Property: The Opposite Sides of a Parallelogram Are of Equal Length., Property: The Opposite Angles of a Parallelogram Are of Equal Measure., Property: The diagonals of a rhombus are perpendicular bisectors of one another..
Using Selina Concise Mathematics [English] Class 9 ICSE solutions Rectilinear Figures [Quadrilaterals: Parallelogram, Rectangle, Rhombus, Square and Trapezium] exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in Selina Solutions are essential questions that can be asked in the final exam. Maximum CISCE Concise Mathematics [English] Class 9 ICSE students prefer Selina Textbook Solutions to score more in exams.
Get the free view of Chapter 14, Rectilinear Figures [Quadrilaterals: Parallelogram, Rectangle, Rhombus, Square and Trapezium] Concise Mathematics [English] Class 9 ICSE additional questions for Mathematics Concise Mathematics [English] Class 9 ICSE CISCE, and you can use Shaalaa.com to keep it handy for your exam preparation.
