Advertisements
Advertisements
Question
In the alongside diagram, ABCD is a parallelogram in which AP bisects angle A and BQ bisects angle B.

Prove that:
- AQ = BP
- PQ = CD
- ABPQ is a parallelogram.
Solution
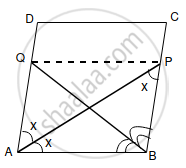
Given: ABCD is a parallelogram and AP bisects ∠A and BQ bisects ∠B.
To prove:
- AQ = BP
- PQ = CD
- ABPQ is a parallelogram.
Proofs:
Let ∠QAB = 2x
∠ABP = 180 - 2x
∠PAB = x
∠APB = 180 - (x + 180 - 2x)
= 180 - (180 - x)
= 180 - 180 + x
= x
In ΔBAP,
∠BAP = ∠APB
or AB = BP ...(1)
Now, ∠PBA = 180 - 2x
∠PBQ = ∠QBA
∠QBA = `(180 - 2x)/2`
= 90 - x
So, ∠PBQ = 90 - x
and ∠PBQ = ∠AQB ...[Alternate Interior Angles]
∠AQB = 90 - x
∠QBA = ∠AQB
hence, AB = AQ ...(2)
From equations (1) & (2) we get
∴ AQ = PB
Since, AQ = BP & ...(As ABCD is Parallelogram)
AQ || PB ...(Opposite sides are equal & parallel, So, ABPQ is a parallelogram)
AB = PQ ...(As ABPQ is a parallelogram)
and AB = CD ...(As ABCD is a parallelogram)
Hence, CD = PQ
APPEARS IN
RELATED QUESTIONS
E is the mid-point of side AB and F is the mid-point of side DC of parallelogram ABCD. Prove that AEFD is a parallelogram.
In the given figure, ABCD is a parallelogram.
Prove that: AB = 2 BC.
Prove that the bisectors of opposite angles of a parallelogram are parallel.
Points M and N are taken on the diagonal AC of a parallelogram ABCD such that AM = CN. Prove that BMDN is a parallelogram.
In a parallelogram ABCD, E is the midpoint of AB and DE bisects angle D. Prove that: BC = BE.
In a parallelogram ABCD, E is the midpoint of AB and DE bisects angle D. Prove that:CE is the bisector of angle C and angle DEC is a right angle
In the given figure, the perimeter of parallelogram PQRS is 42 cm. Find the lengths of PQ and PS.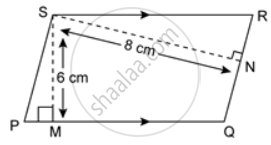
In the Figure, ABCD is a rectangle and EFGH is a parallelogram. Using the measurements given in the figure, what is the length d of the segment that is perpendicular to `bar("HE")` and `bar("FG")`?
In parallelogram ABCD of the accompanying diagram, line DP is drawn bisecting BC at N and meeting AB (extended) at P. From vertex C, line CQ is drawn bisecting side AD at M and meeting AB (extended) at Q. Lines DP and CQ meet at O. Show that the area of triangle QPO is `9/8` of the area of the parallelogram ABCD
In the following figure, ABCD and AEFG are two parallelograms. If ∠C = 55º, determine ∠F.