Advertisements
Advertisements
Question
The following figure shows a trapezium ABCD in which AB is parallel to DC and AD = BC. 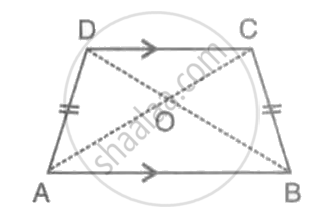
Prove that:
(i) ∠DAB = ∠CBA
(ii) ∠ADC = ∠BCD
(iii) AC = BD
(iv) OA = OB and OC = OD.
Solution
Given ABCD is a trapezium, AB || DC and AD = BC.
Prove that:
(i) ∠DAB = ∠CBA
(ii) ∠ADC = ∠BCD
(iii) AC = BD
(iv) OA = OB and OC = OD.
Proof: (i) Since AD || CB and transversal AC cuts them at A and O respectively.
Therefore, ∠A + ∠B = 180°
Since, AB || CD and AD || BC
Therefore, ABCD is a parallelogram.
∠A = ∠C
∠B = ∠D ....[ Since ABCD is a parallelogram ]
Therefore,
∠DAB = ∠CBA
∠ADC = ∠BCD
In ΔABC and ΔBAD, we have
BC = AD ....( given )
AB = BA ....( Common )
∠A = ∠B ....( proved )
ΔABC ≅ ΔBAD ....( SAS )
ΔABC ≅ ΔBAD
Since, Therefore AC = BD....( Corresponding parts of congruent triangles are equal. )
OA = OB
Again OC = OD ....( Since diagonals bisect each other at O )
Hence proved.
APPEARS IN
RELATED QUESTIONS
E is the mid-point of side AB and F is the mid-point of side DC of parallelogram ABCD. Prove that AEFD is a parallelogram.
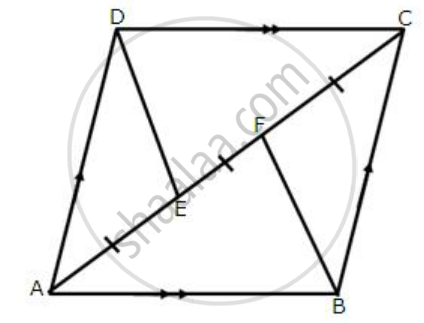
The alongside figure shows a parallelogram ABCD in which AE = EF = FC.
Prove that:
- DE is parallel to FB
- DE = FB
- DEBF is a parallelogram.
In the alongside diagram, ABCD is a parallelogram in which AP bisects angle A and BQ bisects angle B.

Prove that:
- AQ = BP
- PQ = CD
- ABPQ is a parallelogram.
ABCD is a parallelogram. The bisector of ∠BAD meets DC at P, and AD is half of AB.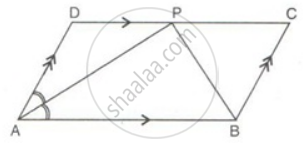
Prove that: BP bisects ∠ABC.
In the given figure, MP is the bisector of ∠P and RN is the bisector of ∠R of parallelogram PQRS. Prove that PMRN is a parallelogram.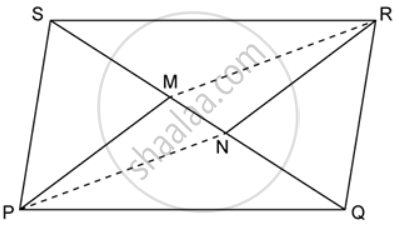
In a parallelogram ABCD, E is the midpoint of AB and DE bisects angle D. Prove that:CE is the bisector of angle C and angle DEC is a right angle
In the given figure, the perimeter of parallelogram PQRS is 42 cm. Find the lengths of PQ and PS.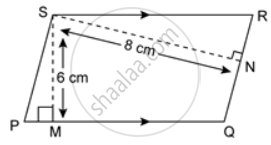
Find the perimeter of the parallelogram PQRS.

Which of the following statement is correct?
In the following figure, ABCD and AEFG are two parallelograms. If ∠C = 55º, determine ∠F.