Advertisements
Advertisements
Question
In case of a parallelogram
prove that:
(i) The bisectors of any two adjacent angles intersect at 90o.
(ii) The bisectors of the opposite angles are parallel to each other.
Solution

ABCD is a parallelogram, the bisectors of ∠ADC and ∠BCD meet at a point E and the bisectors of ∠BCD and ∠ABC meet at F.
We have to prove that the ∠CED = 90° and ∠CFG = 90°
Proof: In the parallelogram ABCD
∠ADC + ∠BCD = 180° ....[ sum of adjacent angles of a parallelogram ]
⇒ `"∠ADC"/2 + "∠BCD"/2` = 90°
⇒ ∠EDC + ∠ECD + ∠CED = 180°
⇒ ∠CED = 90°
Similarly taking triangle BCF it can be proved that ∠BFC = 90°
∠BFC + ∠CFG = 180° ....[ adjacent angles on a line ]
Also ⇒ ∠CFG = 90°
Now since ∠CFG = ∠CED = 90° ....[ It means that the lines DE and BG are parallel ]
Hence proved.
APPEARS IN
RELATED QUESTIONS
In a parallelogram `square`ABCD, If ∠A = (3x + 12)°, ∠B = (2x - 32)° then find the value of x and then find the measures of ∠C and ∠D.
In the following figure, ABCD and PQRS are two parallelograms such that ∠D = 120° and ∠Q = 70°.
Find the value of x.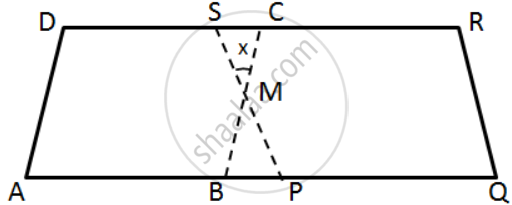
In the following figures, find the remaining angles of the parallelogram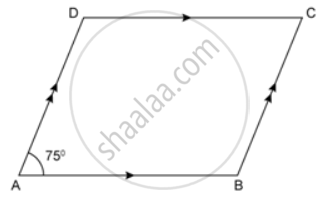
In the following figures, find the remaining angles of the parallelogram
In the following figures, find the remaining angles of the parallelogram
In a parallelogram ABCD ∠C = 98°. Find ∠A and ∠B.
In the given figure, ABCD is a parallelogram, find the values of x and y.
PQR is a triangle formed by the adjacent sides PQ and QR and diagonal PR of a parallelogram PQRS. If in ΔPQR, ∠P : ∠Q : ∠R = 3 : 8 : 4, Calculate the measures of all the angles of parallelogram PQRS.
Opposite angles of a quadrilateral ABCD are equal. If AB = 4 cm, determine CD.
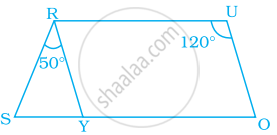
In the given parallelogram YOUR, ∠RUO = 120° and OY is extended to point S such that ∠SRY = 50°. Find ∠YSR.