Advertisements
Advertisements
Question
In a parallelogram `square`ABCD, If ∠A = (3x + 12)°, ∠B = (2x - 32)° then find the value of x and then find the measures of ∠C and ∠D.
Solution

Given: ∠A = (3x + 12)˚ and ∠B = (2x - 32)˚
Opposite angles of a parallelogram are equal.
∴ ∠C = ∠A ...(i)
⇒ ∠C = (3x + 12)˚
∠D = ∠B …(ii)
∠D = (2x - 32)˚
In a quadrilateral, the sum of all the angles is equal to 360˚.
∴ In `square`ABCD,
∠A + ∠B + ∠C + ∠D = 360˚
∴ 3x + 12 + 2x - 32 + 3x + 12 + 2x - 32 = 360
∴ 10x - 40 = 360
∴ 10x = 360 + 40
∴ 10x = 400
∴ x = `400/10`
∴ x = 40
∴ ∠A = (3x + 12)˚
⇒ ∠A = 3 × 40 +12
⇒ ∠A = 120 + 12
⇒ ∠A = 132˚
∴ ∠C = 132˚ ...[From (i)]
∴ ∠B = (2x - 32)˚
⇒ ∠B = 2 × 40 - 32
⇒ ∠B = 80 - 32
⇒ ∠B = 48˚
∴ ∠D = 48˚ ...[From (ii)]
Hence, the measure of x is 40.
Also, measures of ∠C and ∠D are 132˚ and 48˚ respectively.
APPEARS IN
RELATED QUESTIONS
In case of a parallelogram
prove that:
(i) The bisectors of any two adjacent angles intersect at 90o.
(ii) The bisectors of the opposite angles are parallel to each other.
In the given figure, AP is the bisector of ∠A and CQ is the bisector of ∠C of parallelogram ABCD. 
Prove that APCQ is a parallelogram.
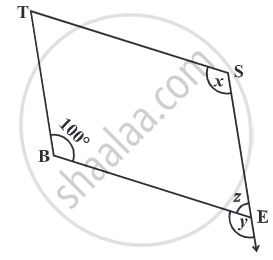
In the following figures, find the remaining angles of the parallelogram
In the following figures, find the remaining angles of the parallelogram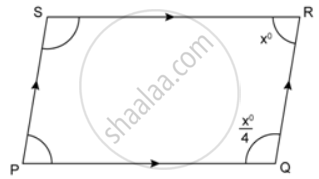
In a parallelogram ABCD ∠C = 98°. Find ∠A and ∠B.
Find the measures of all the angles of the parallelogram shown in the figure: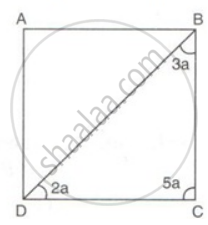
PQR is a triangle formed by the adjacent sides PQ and QR and diagonal PR of a parallelogram PQRS. If in ΔPQR, ∠P : ∠Q : ∠R = 3 : 8 : 4, Calculate the measures of all the angles of parallelogram PQRS.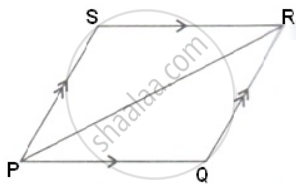
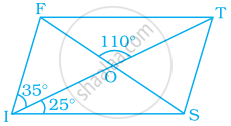
In parallelogram FIST, find ∠SFT, ∠OST and ∠STO.
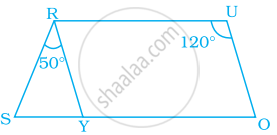
In the given parallelogram YOUR, ∠RUO = 120° and OY is extended to point S such that ∠SRY = 50°. Find ∠YSR.