Advertisements
Advertisements
Question
In the following figures, find the remaining angles of the parallelogram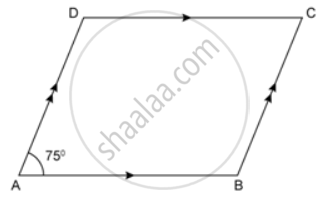
Solution
ABCD is a parallelogram.
∠A = 75°
⇒ ∠C = 75° ....(Opposite angles of a parallelogram are eequal)
Now,∠A + ∠D = 180° ....(Interior angles)
⇒ ∠75° + ∠D = 180°
⇒ ∠D = 105°
⇒ ∠B = ∠D = 105° ....(Opposite angles of a parallelogram are equal)
Thus, we have
∠B = 105°, ∠C = 75° and ∠D = 105°.
APPEARS IN
RELATED QUESTIONS
In a parallelogram `square`ABCD, If ∠A = (3x + 12)°, ∠B = (2x - 32)° then find the value of x and then find the measures of ∠C and ∠D.
In the following figure, ABCD and PQRS are two parallelograms such that ∠D = 120° and ∠Q = 70°.
Find the value of x.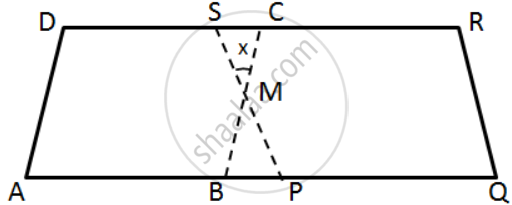
In the given figure, AP is the bisector of ∠A and CQ is the bisector of ∠C of parallelogram ABCD. 
Prove that APCQ is a parallelogram.
In the following figures, find the remaining angles of the parallelogram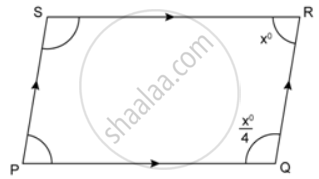
Find the measures of all the angles of the parallelogram shown in the figure: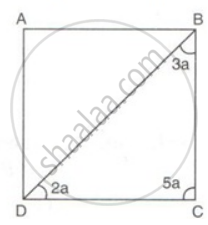
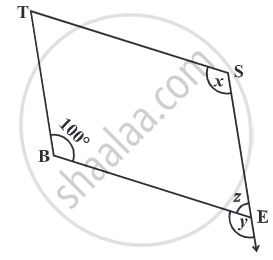
Opposite angles of a quadrilateral ABCD are equal. If AB = 4 cm, determine CD.
The sum of adjacent angles of a parallelogram is ______.
In parallelogram FIST, find ∠SFT, ∠OST and ∠STO.
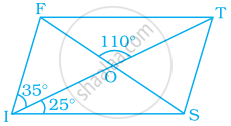
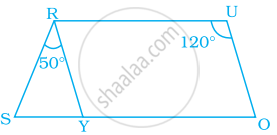
In the given parallelogram YOUR, ∠RUO = 120° and OY is extended to point S such that ∠SRY = 50°. Find ∠YSR.