Advertisements
Advertisements
Question
In the given figure ABCD is a rhombus with angle A = 67°

If DEC is an equilateral triangle, calculate:
- ∠CBE
- ∠DBE
Solution
Given that the figure ABCD is a rhombus with angle A = 67o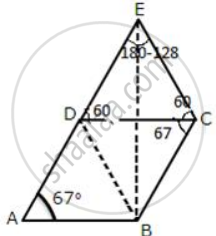
In the rhombus we have
∠A = 67° = ∠C ...[Opposite angles]
∠A + ∠D = 180° ...[Consecutive angles are supplementary.]
⇒ ∠D = 113°
⇒ ∠ABC = 113°
Consider ΔDBC,
DC = CB ...[Sides of rhombus]
So, ΔDBC is an isosceles triangle,
⇒ ∠CDB = ∠CBD
Also,
∠CDB + ∠CBD + ∠BCD = 180°
⇒ 2∠CBD = 113°
⇒ ∠CDB = ∠CBD = 56.5° ...(i)
Consider ΔDCE,
EC = CB
So ΔBCE is an isosceles triangle
⇒ ∠CBE = ∠CEB
Also,
∠CBE + ∠CEB + ∠BCE = 180°
⇒ 2∠CBE = 53°
⇒ ∠CDE = 26.5°
From (i)
∠CBD = 56.5°
⇒ ∠CBE + ∠DBE = 56.5°
⇒ 26.5° + ∠DBE = 56.5°
⇒ ∠DBE = 56.5° - 26.5°
⇒ ∠DBE = 30°
APPEARS IN
RELATED QUESTIONS
Show that the diagonals of a square are equal and bisect each other at right angles.
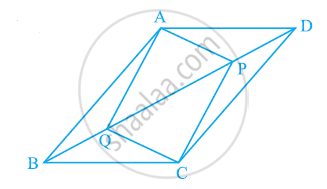
In parallelogram ABCD, two points P and Q are taken on diagonal BD such that DP = BQ (see the given figure). Show that:
- ΔAPD ≅ ΔCQB
- AP = CQ
- ΔAQB ≅ ΔCPD
- AQ = CP
- APCQ is a parallelogram
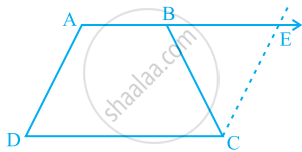
ABCD is a trapezium in which AB || CD and AD = BC (see the given figure). Show that
- ∠A = ∠B
- ∠C = ∠D
- ΔABC ≅ ΔBAD
- diagonal AC = diagonal BD
[Hint: Extend AB and draw a line through C parallel to DA intersecting AB produced at E.]
State, 'true' or 'false'
The diagonals of a quadrilateral bisect each other.
State, 'true' or 'false'
The quadrilateral, whose four sides are equal, is a square.
State, 'true' or 'false'
Every rhombus is a parallelogram.
State, 'true' or 'false'
Diagonals of a rhombus are equal.
In the following figures, ABCD is a parallelogram.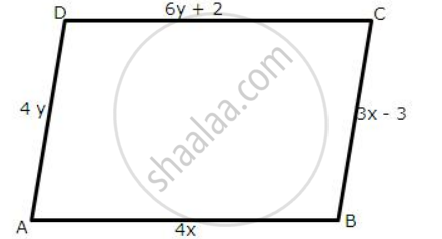
find the values of x and y.
In the figure, given below, AM bisects angle A and DM bisects angle D of parallelogram ABCD. Prove that: ∠AMD = 90°.

In a square ABCD, diagonals meet at O. P is a point on BC such that OB = BP.
Show that:
- ∠POC = `[ 22 ( 1°)/( 2 ) ]`
- ∠BDC = 2 ∠POC
- ∠BOP = 3 ∠CPO
ABCD is a parallelogram having an area of 60cm2. P is a point on CD. Calculate the area of ΔAPB.
In the given figure area of ∥ gm PQRS is 30 cm2. Find the height of ∥ gm PQFE if PQ = 6 cm.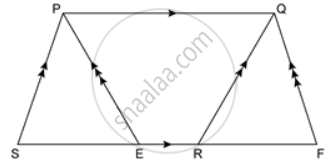
In the given figure, PT ∥ QR and QT ∥ RS. Show that: area of ΔPQR = area of ΔTQS.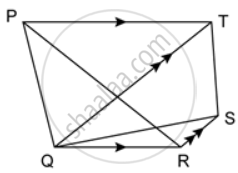
*Question modified
Find the area of each of the following figure: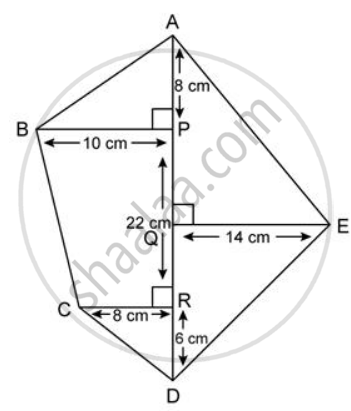
Two adjacent sides of a parallelogram are 34 cm and 20 cm. If one of its diagonal is 42 cm, find: distance between its shorter sides
Find the perimeter and area of a rectangle whose length and breadth are 12cm and 9cm respectively.
PQRS is a square with each side 6cm. T is a point on QR such that the `"area of ΔPQT"/"area of trapezium PTRS" = (1)/(3)` Find the length of TR.
In quadrilateral ABCD, ∠A + ∠D = 180º. What special name can be given to this quadrilateral?
The diagonals of a square are perpendicular to one another.
