Advertisements
Advertisements
Question
In the given figure; ABC, AEQ and CEP are straight lines. Show that ∠APE and ∠CQE are supplementary.
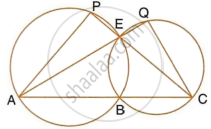
Solution

Given – In the figure, ABC, AEQ and CEP are straight lines
To prove – ∠APE + ∠CQE = 180°
Construction – Join EB
Proof – In cyclic quad ABEP,
∠APE + ∠ABE = 180° ...(1)
Similarly, in cyclic quad BCQE
∠CQE + ∠CBE = 180° ...(2)
Adding (1) and (2),
∠APE + ∠ABE + ∠CQE + ∠CBE = 180° + 180° = 360°
`=>` ∠APE + ∠CQE + ∠ABE + ∠CBE = 360°
But, ∠ABE + ∠CBE = 180° ...[Linear pair]
∴ ∠APE + ∠CQE + 180° = 360°
`=>` ∠APE + ∠CQE = 360° – 180° = 180°
Hence ∠APE and ∠CQE are supplementary.
APPEARS IN
RELATED QUESTIONS
In the given figure, two circles touch each other externally at point P. AB is the direct common tangent of these circles. Prove that :

- tangent at point P bisects AB,
- angles APB = 90°.
In the given figure, two circles touch each other externally at point P. AB is the direct common tangent of these circles. Prove that:

(ii) angles APB = 90°
Two parallel tangents of a circle meet a third tangent at points P and Q. Prove that PQ subtends a right angle at the centre.
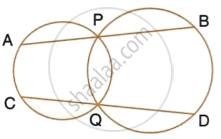
Two circles intersect at P and Q. Through P, a straight line APB is drawn to meet the circles in A and B. Through Q, a straight line is drawn to meet the circles at C and D. Prove that AC is parallel to BD.
Two circles intersect each other at points A and B. Their common tangent touches the circles at points P and Q as shown in the figure. Show that the angles PAQ and PBQ are supplementary.

Radius of a sector of a circle is 21 cm. If length of arc of that sector is 55 cm, find the area of the sector.
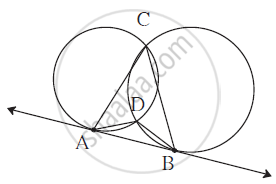
Two circles intersect each other at points C and D. Their common tangent AB touches the circles at point A and B. Prove that :
∠ ADB + ∠ ACB = 180°
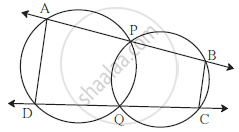
Two circles intersect each other at points P and Q. Secants drawn through P and Q intersect the circles at points A,B and D,C. Prove that : ∠ADC + ∠BCD = 180°
Two circles of radii 5cm and 3cm with centres O and P touch each other internally. If the perpendicular bisector of the line segment OP meets the circumference of the larger circle at A and B, find the length of AB.
P and Q are the centre of circles of radius 9 cm and 2 cm respectively; PQ = 17 cm. R is the centre of circle of radius x cm, which touches the above circles externally, given that ∠ PRQ = 90°. Write an equation in x and solve it.
