Advertisements
Advertisements
Question
In the given figure, HT shows the height of a tree standing vertically. From a point P, the angle of elevation of the top of the tree (that is ∠P) measures 42° and the distance to the tree is 60 metres. Find the height of the tree
Solution
Let the height of the tree HT be “x”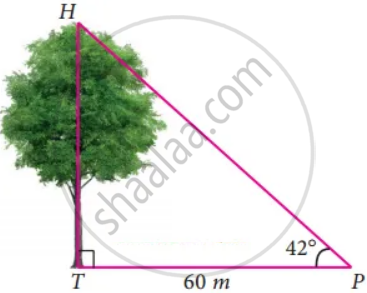
In the ΔHTP,
tan 42° = `"HT"/"PT"`
0.9004 = `x/60`
x = 0.9004 × 60
= 54.024
The height of the tree = 54.02 m
APPEARS IN
RELATED QUESTIONS
In the following table, a ratio is given in each column. Find the remaining two ratios in the column and complete the table.
| sin θ | `11/61` | `1/2` | `3/5` | ||||||
| cos θ | `35/37` | `1/sqrt3` | |||||||
| tan θ | `1` | `21/20` | `8/15` | `1/(2sqrt2)` |
Find the value of the following:
cos 74° 39′
Find the value of the following:
cos 33° 53′
Find the value of θ if cos θ = 0.6763
Find the value of θ if tan θ = 0.0720
Find the value of θ if cos θ = 0.0410
Find the value of the following:
sin 65° 39′ + cos 24° 57’ + tan 10° 10′
Find the value of the following:
tan 70° 58′ + cos 15° 26′ – sin 84° 59′
Find the angle made by a ladder of length 5m with the ground, if one of its end is 4m away from the wall and the other end is on the wall
The value of 2tan45° – 2sin30° is ______.
