Advertisements
Advertisements
Question
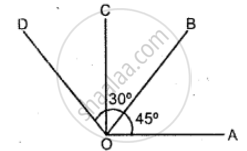
In the given figure:
(i) If ∠AOB = 45°, ∠BOC = 30° and ∠AOD= 110°; find : angles COD and BOD.
(ii) If ∠BOC = ∠DOC = 34° and ∠AOD = 120° ; find : angle AOB and angle AOC.
(iii) If ∠AOB = ∠BOC = ∠COD = 38° find : reflex angle AOC and reflex angle AOD.
Solution
(i) ∠COD = ∠AOD – ∠AOC
= ∠AOD – (∠AOB + ∠BOC)
= 110° - (45°+ 30°)
= 110°- 75°
= 35°
∠BOD = ∠AOD -∠AOB
= 110° - 45°
= 65°
(ii) ∠AOB = ∠AOD-∠BOD
= ∠AOD – (∠BOC + ∠COD)
= 120° – (34° + 34°)
= 120°-68°
= 52°
∠AOC = ∠AOB + ∠BOC
= 52° + 34°
= 86°
(iii) Reflex ∠AOC = 360°-∠AOC
= 360° – (∠AOB + ∠BOC)
= 360° – (38° + 38°)
= 360° – 76° = 284°
Reflex ∠AOD = 360° – ∠AOD
= 360° (∠AOB + ∠BOC + ∠COD)
= 360° – (38° + 38° + 38°)
= 360°- 114°
= 246°
APPEARS IN
RELATED QUESTIONS
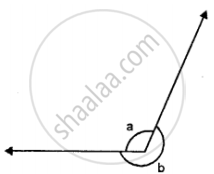
In the given figure if: b = 200 ; find a.
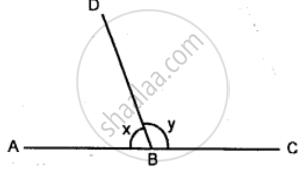
In the given diagram, ABC is a straight line. If y =`1 1/2` right angles; find x.
Write the supplement angle of: 20° + y°
Write the complement angle of: `1/6` of 90°
Find the angle that is equal to its supplement?
For an angle x°, find:
(i) the complementary angle
(ii) the supplementary angle
(iii) the value of x° if its supplementary angle is three times its complementary angle.
Explain what do you understand by Adjacent angles?
Explain what do you understand by complementary angles?
Find the angle formed by the arms of a clock at 6 O’clock.
Find the angle formed by the arms of a clock at 12 O’clock.
