Advertisements
Advertisements
प्रश्न
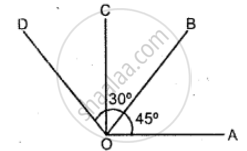
In the given figure:
(i) If ∠AOB = 45°, ∠BOC = 30° and ∠AOD= 110°; find : angles COD and BOD.
(ii) If ∠BOC = ∠DOC = 34° and ∠AOD = 120° ; find : angle AOB and angle AOC.
(iii) If ∠AOB = ∠BOC = ∠COD = 38° find : reflex angle AOC and reflex angle AOD.
उत्तर
(i) ∠COD = ∠AOD – ∠AOC
= ∠AOD – (∠AOB + ∠BOC)
= 110° - (45°+ 30°)
= 110°- 75°
= 35°
∠BOD = ∠AOD -∠AOB
= 110° - 45°
= 65°
(ii) ∠AOB = ∠AOD-∠BOD
= ∠AOD – (∠BOC + ∠COD)
= 120° – (34° + 34°)
= 120°-68°
= 52°
∠AOC = ∠AOB + ∠BOC
= 52° + 34°
= 86°
(iii) Reflex ∠AOC = 360°-∠AOC
= 360° – (∠AOB + ∠BOC)
= 360° – (38° + 38°)
= 360° – 76° = 284°
Reflex ∠AOD = 360° – ∠AOD
= 360° (∠AOB + ∠BOC + ∠COD)
= 360° – (38° + 38° + 38°)
= 360°- 114°
= 246°
APPEARS IN
संबंधित प्रश्न
In the given figure if: b = 200 ; find a.
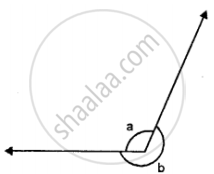
In the given figure if: a = 5/3 right angle, find b

In the given diagram, ABC is a straight line. If x = 53°, find y.

In the given figure, lines AB and CD intersect at point O.
(i) Find the value of ∠a.
(ii) Name all the pairs of vertically opposite angles.
(iii) Name all the pairs of adjacent angles.
(iv) Name all the reflex angles formed and write the measure of each.

Write the complement angle of: 20° + y°
Two supplementary angles are in the ratio 7 : 11. Find the angles.
The measures of two supplementary angles are (3x + 15)° and (2x + 5)°. Find x.
Find the angle formed by the arms of a clock at 3 O’clock.
Find the angle formed by the arms of a clock at 6 O’clock.
An angle is one-thirds of a straight line angle; find:
(i) the angle
(ii) the complement and the supplement of the angle obtained above.
