Advertisements
Advertisements
Question
In the given figure, ratio of the area of triangle ABC to the area of triangle ACD is the same as the ratio of base BC of triangle ABC to the base CD of ΔACD.
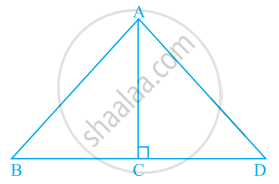
Options
True
False
Solution
This statement is True.
Explanation:

∵ Area of ΔABC : Area of ΔACD = `1/2` × BC × AC : `1/2` × CD × AC ......[∵ Area of triangle = Base × Height]
= BC : CD
APPEARS IN
RELATED QUESTIONS
Find the area of the following triangle:

Prove that the lines joining the middle points of the opposite sides of a quadrilateral and the join of the middle points of its diagonals meet in a point and bisect one another
If the coordinates of the mid-points of the sides of a triangle are (1, 1), (2, —3) and (3, 4), find the vertices of the triangle.
Find the area of ΔABC with A(1, -4) and midpoints of sides through A being (2, -1) and (0, -1).
Using integration, find the area of triangle ABC, whose vertices are A(2, 5), B(4, 7) and C(6, 2).
If the points (a1, b1), (a2, b2) and(a1 + a2, b1 + b2) are collinear, then ____________.
Find the coordinates of the point Q on the x-axis which lies on the perpendicular bisector of the line segment joining the points A(–5, –2) and B(4, –2). Name the type of triangle formed by the points Q, A and B.
The base and the corresponding altitude of a parallelogram are 10 cm and 3.5 cm, respectively. The area of the parallelogram is 30 cm2.
The area of a trapezium is 475 cm2 and the height is 19 cm. Find the lengths of its two parallel sides if one side is 4 cm greater than the other.
Triangles having the same base have equal area.
