Advertisements
Advertisements
Question
In the natural numbers from 10 to 250, how many are divisible by 4?
Solution
The least positive natural number from 10 to 250 divisible by 4 is 12.
The sequence divisible by 4 is 12, 16, 20, .... , 248.
Now,
\[t_n = a + \left( n - 1 \right)d\]
\[ \Rightarrow 248 = 12 + \left( n - 1 \right)\left( 4 \right)\]
\[ \Rightarrow 248 = 12 + 4n - 4\]
\[ \Rightarrow 248 = 8 + 4n\]
\[ \Rightarrow 248 - 8 = 4n\]
\[ \Rightarrow 4n = 240\]
\[ \Rightarrow n = 60\]
APPEARS IN
RELATED QUESTIONS
For a sequence if n `S_n=n/(n+1)` then find the value of S10.
Find the first two terms of the sequence for which Sn is given below: `S_n=n^2(n+1)`
How many three digit natural numbers are divisible by 5?
Find how many three digit natural numbers are divisible by 5.
Complete the following activity to find the sum of natural numbers from 1 to 140 which are divisible by 4.
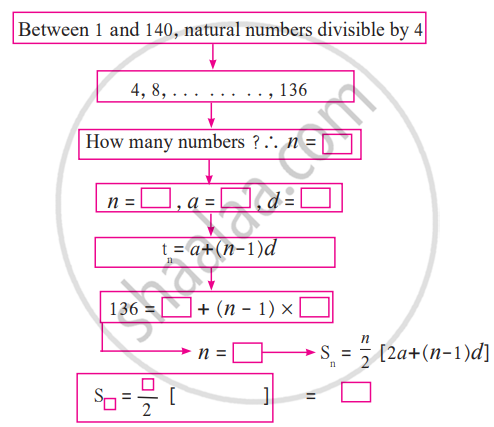
Sum of numbers from 1 to 140, which are divisible by 4 = `square`
Find the fourth term from the end in an A.P. –11, –8, –5,...., 49.
Solve any four of the following.
Write the set Q of rational numbers in the property form.
Solve any two of the following.
If a : b = 7 : 2, find the ratios (i)` b : a` (ii) `(a+b)/b`
Multiply and write the answer in the simplest form :
`5sqrt7 xx 2sqrt7`
Find the first three terms to the sequence, whose nth term is tn = 4n - 3
q1
Choose the correct alternative answer for the following sub questions
A set of numbers where the numbers are arranged in a definite order, like the natural numbers, is called a ______
There is an auditorium with 27 rows of seats. There are 20 seats in the first row, 22 seats in the second row, 24 seats in the third row and so on. Find how many total seats are there in the auditorium?
