Advertisements
Advertisements
Question
Obtain the expression for the energy stored per unit volume in a charged parallel plate capacitor.
Advertisements
Solution
Let us consider a parallel-plate capacitor of plate area A
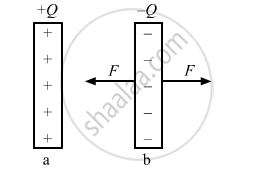
Suppose the plates of the capacitor are almost touching each other and a charge Q is given to the capacitor. One of the plates, say a, is kept fixed and other say b, is slowly pulled away from plate a to increase the separation from zero to d. The attractive force on the plate b at any instant due to first plate is given by:
`F=Q^2/(2Aepsilon_0)`
The person pulling the plate b must apply an equal and opposite force (F) in the opposite direction if the plate is moved slowly.
Work done by the person during the displacement of the second plate:
W = Fd
`=(Q^2d)/(2Aepsilon_0)=Q^2/(2C)`
Here,`C=(Aepsilon_0)/d`, which is the capacitance of the capacitor in the final position.
The work done by the person must be equal to the increase in the energy of the system.
Thus, the capacitor has a stored energy.
`U=Q^2/(2C)`
Now, if we pull the plates of the capacitor apart, we have to do work against the electrostatic attraction between the plates. When we increase the separation between the plates from d1 to d2, an amount `Q^2/(2Aepsilon_0)(d_2-d_1)` of work is performed by us and this much energy goes into the capacitor. On the other hand, new electric field is created in a volume A(d2 - d1).
The energy stored per unit volume is thus given by:
`u=((Q^2(d_2-d_1))/(2Aepsilon_0))/(A(d_2-d_1))=Q^2/(2A^2epsilon_0)`
`1/2epsilon_0(Q/(Aepsilon_0))^2=1/2epsilon_0E^2`
Here, E is the intensity of the electric field.
APPEARS IN
RELATED QUESTIONS
Find the ratio of energy stored in the two configurations if they are both connected to the same source.
A capacitor C1 of capacitance 1 μF and a capacitor C2 of capacitance 2 μF are separately charged by a common battery for a long time. The two capacitors are then separately discharged through equal resistors. Both the discharge circuits are connected at t = 0.
(a) The current in each of the two discharging circuits is zero at t = 0.
(b) The currents in the two discharging circuits at t = 0 are equal but not zero.
(c) The currents in the two discharging circuits at t = 0 are unequal.
(d) C1 loses 50% of its initial charge sooner than C2 loses 50% of its initial charge.
How many time constants will elapse before the current in a charging RC circuit drops to half of its initial value? Answer the same question for a discharging RC circuit.
A capacitor of capacitance 12.0 μF is connected to a battery of emf 6.00 V and internal resistance 1.00 Ω through resistanceless leads. 12.0 μs after the connections are made, what will be (a) the current in the circuit (b) the power delivered by the battery (c) the power dissipated in heat and (d) the rate at which the energy stored in the capacitor is increasing?
A capacitor with stored energy 4⋅0 J is connected with an identical capacitor with no electric field in between. Find the total energy stored in the two capacitors.
A capacitor of capacitance C is given a charge Q. At t = 0, it is connected to an ideal battery of emf ε through a resistance R. Find the charge on the capacitor at time t.
An air-filled parallel plate capacitor has a uniform electric field `overset(->)("E")` in the space between the plates. If the distance between the plates is 'd' and the area of each plate is 'A', the energy stored in the capacitor is ______
(∈0 = permittivity of free space)
What fraction of the energy drawn from the charging battery is stored in a capacitor?
In a capacitor of capacitance 20 µF, the distance between the plates is 2 mm. If a dielectric slab of width 1 mm and dielectric constant 2 is inserted between the plates, what is the new capacitance?
If the charge on the parallel plate capacitor is increased by 3 C, the energy stored in it increases by 44%. The original charge on the capacitor is ______.
