Advertisements
Advertisements
Question
In a series LCR circuit connected to an a.c. source of voltage v = vmsinωt, use phasor diagram to derive an expression for the current in the circuit. Hence, obtain the expression for the power dissipated in the circuit. Show that power dissipated at resonance is maximum
Solution
The phasor diagram for the series LCR circuit is

The phasor relation for the voltages is
VL + VR + VC = V
Because VC and VL are always along the same line and in opposite directions, they can be combined into a single phasor (VC + VL) which has a magnitude |vCm - vLm|.
Because V is represented as the hypotenuse of a right triangle whose sides are VR and (VC + VL), the Pythagorean theorem gives
`v_m^2=v_(Rm)^2+(V_(Cm)-V_(Lm))^2`
`:.v_m^2=(i_mR)^2+(i_mX_c-i_mX_L)^2`
`:.v_m^2=i_m^2[R^2+(X_C-X_L)^2]`
`:.i_m=v_m/sqrt(R^2+(X_C-X_L)^2)`
This is the expression for the current in the circuit.
The instantaneous power in the circuit is
P = vi = (vmsinωt)(imsin(ωt+Φ))
`:. P=(v_mi_m)/2(sinomegat)(sin(omegat+phi))`
`:.P=(v_mi_m)/2(cosphi-cos(2omegat+phi))`
The average power over a cycle is given by the average of the two terms in the RHS of the above equation.
It is only the second term (cos(2ωt+)) which is time-dependent. Its average is zero. Therefore, we get
`P=(v_mi_m)/2cosphi=v_m/sqrt2xxi_m/sqrt2cosphi`
∴ P = VI cosΦ
It can also be written as P = I2ZcosΦ
At resonance XC − XL = 0 and Φ = 0. Therefore, cosΦ = 1 and power is P=I2Z=I2R.
That is, maximum power is dissipated in a circuit at resonance.
APPEARS IN
RELATED QUESTIONS
In a series LCR circuit, obtain the condition under which the impedance of the circuit is minimum ?
A series LCR circuit is connected to a source having voltage v = vm sin ωt. Derive the expression for the instantaneous current I and its phase relationship to the applied voltage.
Obtain the condition for resonance to occur. Define ‘power factor’. State the conditions under which it is (i) maximum and (ii) minimum.
Derive an expression for the average power consumed in a series LCR circuit connected to a.c. source in which the phase difference between the voltage and the current in the circuit is Φ.
The time constant of an LR circuit is 40 ms. The circuit is connected at t = 0 and the steady-state current is found to be 2.0 A. Find the current at (a) t = 10 ms (b) t = 20 ms, (c) t = 100 ms and (d) t = 1 s.
In a series LCR circuit the voltage across an inductor, capacitor and resistor are 20 V, 20 V and 40 V respectively. The phase difference between the applied voltage and the current in the circuit is ______.
At resonant frequency the current amplitude in series LCR circuit is ______.
A series LCR circuit contains inductance 5 mH, capacitance 2µF and resistance ion. If a frequency A.C. source is varied, what is the frequency at which maximum power is dissipated?
In series LCR AC-circuit, the phase angle between current and voltage is
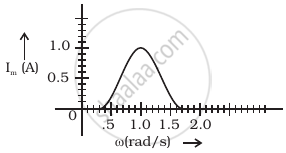
In series LCR circuit, the plot of Imax vs ω is shown in figure. Find the bandwidth and mark in the figure.
Draw the impedance triangle for a series LCR AC circuit and write the expressions for the impedance and the phase difference between the emf and the current.
