Advertisements
Advertisements
Question
Prove that the diagonals of a rectangle ABCD with vertices A(2,-1), B(5,-1) C(5,6) and D(2,6) are equal and bisect each other
Solution
The vertices of the rectangle ABCD are A(2, -1), B(5, -1), C(5, 6) and D(2, 6) Now,
`"Coordinates of midpoint of" AC = ((2+5)/2 , (-1+6)/2) = (7/5 ,5/2)`
`"Coordinates of midpoint of " BD = ((5+2)/2 , (-1+6)/2)= (7/2,5/2)`
Since, the midpoints of AC and BD coincide, therefore the diagonals of rectangle ABCD bisect each other.
APPEARS IN
RELATED QUESTIONS
Let ABCD be a square of side 2a. Find the coordinates of the vertices of this square when The centre of the square is at the origin and coordinate axes are parallel to the sides AB and AD respectively.
Show that the points A(5, 6), B(1, 5), C(2, 1) and D(6,2) are the vertices of a square.
Find the points of trisection of the line segment joining the points:
5, −6 and (−7, 5),
Find the points of trisection of the line segment joining the points:
(3, -2) and (-3, -4)
Prove that the points A(-4,-1), B(-2, 4), C(4, 0) and D(2, 3) are the vertices of a rectangle.
Find the ratio in which the pint (-3, k) divide the join of A(-5, -4) and B(-2, 3),Also, find the value of k.
The perpendicular distance of the P (4,3) from y-axis is
Write the ratio in which the line segment doining the points A (3, −6), and B (5, 3) is divided by X-axis.
If P (2, p) is the mid-point of the line segment joining the points A (6, −5) and B (−2, 11). find the value of p.
Find the area of triangle with vertices ( a, b+c) , (b, c+a) and (c, a+b).
If the points (k, 2k), (3k, 3k) and (3, 1) are collinear, then k
If the area of the triangle formed by the points (x, 2x), (−2, 6) and (3, 1) is 5 square units , then x =
The length of a line segment joining A (2, −3) and B is 10 units. If the abscissa of B is 10 units, then its ordinates can be
If the line segment joining the points (3, −4), and (1, 2) is trisected at points P (a, −2) and Q \[\left( \frac{5}{3}, b \right)\] , Then,
What is the form of co-ordinates of a point on the X-axis?
Write the equations of the x-axis and y-axis.
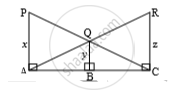
In the above figure, seg PA, seg QB and RC are perpendicular to seg AC. From the information given in the figure, prove that: `1/x + 1/y = 1/z`
A point both of whose coordinates are negative will lie in ______.
Assertion (A): The point (0, 4) lies on y-axis.
Reason (R): The x-coordinate of a point on y-axis is zero.
Distance of the point (6, 5) from the y-axis is ______.
