Advertisements
Advertisements
Question
Show that gravity decreases at higher altitudes.
Solution
We know that the law of gravitation is
F = G ( m1 x m2)/R2
Here the F is the force due to attraction and this force is equal to the weight of the body m2g.
So m2g = G ( m1 x m2)/R2
g= (G x m2)/R2.
Here R is the distance between the earth centre and the object centre. Now if we go on higher altitude say ‘H’ then this R would increase to (R + H)
And the value of gravity at height H becomes
g’= (G x m2)/( R + H)2.
As denominator increases so g’ would be less than g and hence we can say that gravity decreases at higher altitudes.
APPEARS IN
RELATED QUESTIONS
Write the answer of the question with reference to laws of gravitation.
State the universal law of gravitation.
Calculate the force of gravitation between the earth and the Sun, given that the mass of the earth = 6 × 1024 kg and of the Sun = 2 × 1030 kg. The average distance between the two is 1.5 × 1011 m.
At noon, the sun and the earth pull the objects on the earth's surface in opposite directions. At midnight, the sun and the earth pull these objects in same direction. Is the weight of an object, as measured by a spring balance on the earth's surface, more at midnight as compared to its weight at noon?
A person sitting in a chair in a satellite feels weightless because
Four particles of equal masses M move along a circle of radius R under the action of their mutual gravitational attraction. Find the speed of each particle.
The law of gravitation gives the gravitational force between :
Is the law of gravitation applicable in case of the sun and the moon?
A force can produce ________, In an object at rest. It can __________ an object and change its __________ of motion.
For the weight of body of mass 5 kg to be zero on equator of the earth, angular velocity of the earth must be (The radius of earth = 6400 km, acceleration due to gravity = 10 m/s2).
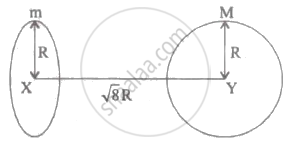
Find the gravitational force of attraction between the ring and sphere as shown in the diagram, where the plane of the ring is perpendicular to the line joining the centres. If `sqrt8` R is the distance between the centres of a ring (of mass 'm')and a sphere (mass 'M') where both have equal radius 'R'.