Advertisements
Advertisements
Question
The figure shows the plot of PV/T versus Pfor 1.00×10–3 kg of oxygen gas at two different temperatures.
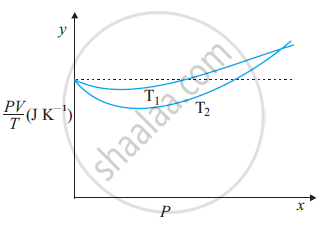
(a) What does the dotted plot signify?
(b) Which is true: T1 > T2 or T1 < T2?
(c) What is the value of PV/T where the curves meet on the y-axis?
(d) If we obtained similar plots for 1.00 ×10–3 kg of hydrogen, would we get the same value of PV/T at the point where the curves meet on the y-axis? If not, what mass of hydrogen yields the same value of PV/T (for low pressure high temperature region of the plot)? (Molecular mass of H2 = 2.02 u, of O2 = 32.0 u, R = 8.31 J mo1–1 K–1.)
Solution 1
a) The dotted plot in the graph signifies the ideal behaviour of the gas, i.e., the ratio `"PV"/T` is equal. μR (μ is the number of moles and R is the universal gas constant) is a constant quality. It is not dependent on the pressure of the gas.
b) The dotted plot in the given graph represents an ideal gas. The curve of the gas at temperature T1 is closer to the dotted plot than the curve of the gas at temperature T2. A real gas approaches the behaviour of an ideal gas when its temperature increases. Therefore, T1 > T2 is true for the given plot.
c) The value of the ratio PV/T, where the two curves meet, is μR. This is because the ideal gas equation is given as:
PV = μRT
Where,
P is the pressure
T is the temperature
V is the volume
μ is the number of moles
R is the universal constant
Molecular mass of oxygen = 32.0 g
Mass of oxygen = 1 × 10–3 kg = 1 g
R = 8.314 J mole–1 K–1
`:. PV/T = 1/32 xx 8.314`
= 0.26 `J K^(-1)`
Therefore, the value of the ratio PV/T, where the curves meet on the y-axis, is 0.26 J K–1.
d) If we obtain similar plots for 1.00 × 10–3 kg of hydrogen, then we will not get the same value of PV/T at the point where the curves meet the y-axis. This is because the molecular mass of hydrogen (2.02 u) is different from that of oxygen (32.0 u).
We have:
`PV/T = 0.26 J K^(-1)`
R = 8.314 J mole–1 K–1
Molecular mass (M) of H2 = 2.02 u
`PV/T = muR` at constant temperature
Where, `mu = m/M`
`m = "Mass of " H_2`
`:.m = (PV)/T xx M/R`
`= (0.26 xx 2.02)/8.31`
= 6.3 × 10–2 g = 6.3 × 10–5 kg
Hence, 6.3 × 10–5 kg of H2 will yield the same value of PV/T.
Solution 2
a) The dotted plot corresponds to ‘ideal’ gas behaviour as it is parallel to P-axis and it tells that value of PV/T remains same even when P is changed.
b) The upper position of PV/T shows that its value is lesser for T1 thus T1 > T2. This is because the curve at T1is more close to dotted plot than the curve at T2 Since the behaviour of a real gas approaches the perfect gas behaviour, as the temperature is increased.
c) Where the two curves meet, the value of PV/T on y-axis is equal to μR. Since ideal gas equation for μ moles is PV = μRT
Where `mu = (1.00 xx 10^(-3) kg)/(32xx10^(-3)kg) = 1/32`
`:. "Value of " "PV"/T = muR = 1/32 xx 8.31 JK^(-1) = 0.26 JK^(-1)`
d) if we obtained similar plots for 1.00 xx 10^(-3) kg of hydrogen, we will not get the same value of `"PV"/T` at the point, where the curves meet of the y-axis. This is because molecular mass of hydrogen is different form that of oxygen.
For the same value of `PV/T`, mass of hydrogen required is obtained from
`"PV"/T = nR = m/2.02 xx 8.31 = 0.26`
`m = (2.02 xx 0.26)/ 8.31 " gram" = 6.32 xx 10^(-2) "gram"`
APPEARS IN
RELATED QUESTIONS
At what temperature is the root mean square speed of an atom in an argon gas cylinder equal to the rms speed of a helium gas atom at – 20 °C? (atomic mass of Ar = 39.9 u, of He = 4.0 u).
Oxygen is filled in a closed metal jar of volume 1.0 × 10−3 m3 at a pressure of 1.5 × 105Pa and temperature 400 K. The jar has a small leak in it. The atmospheric pressure is 1.0 × 105 Pa and the atmospheric temperature is 300 K. Find the mass of the gas that leaks out by the time the pressure and the temperature inside the jar equalise with the surrounding.
What do you understand by gas?
What is diffusion? Give an example to illustrate it.
Give reasons for the following:
Gas fills the vessel completely in which it is kept.
A gas occupies 500 cm3 at a normal temperature. At what temperature will the volume of the gas be reduced by 20% of its original volume, the pressure is constant?
Name or state the following:
An equation used in chemical calculations which gives a simultaneous effect of changes of temperature and pressure on the volume of a given mass of dry gas
Name or state the following:
The standard pressure of a gas in cm. of mercury corresponding to one atmospheric pressure.
Name or state the following:
The absolute temperature value corresponding to 35°C.
For a wave, y = 0.0002 sin`[2pi(110"t"-x/3)+pi/3]` is travelling in a medium. The energy per unit volume being transferred by wave if density of medium is 1.5 kg/m3, is ______.
