Advertisements
Advertisements
Question
Solve the following system of linear equations graphically :
4x - 5y - 20 = 0
3x + 3y - 15 = 0
Determine the vertices of the triangle formed by the lines, represented by the above equations and the y-axis.
Solution
4x - 5y - 20 = 0 ...(1)
3x + 3y - 15 = 0 ...(2)
4x - 5y - 20 = 0
⇒ 4x = 5y + 20
Corresponding values of x and y can be tabulated as :
| x | 0 | -5 | 5 |
| y | -4 | -8 | 0 |
Plotting points (0, -4), (-5, -8), (5, 0) and joining them, we get a line l1 which is the graph of equation (1).
Again, 3x + 3y - 15 = 0
⇒ x + y - 5 = 0
⇒ x+ y = 5
Corresponding values of x and y can be tabulated as :
| x | 0 | -5 | 5 |
| y | -4 | -8 | 0 |
Plotting points (0, 5), (5, 0), (2, 3) and joining them, we get a line l2 which is the graph of equation (2).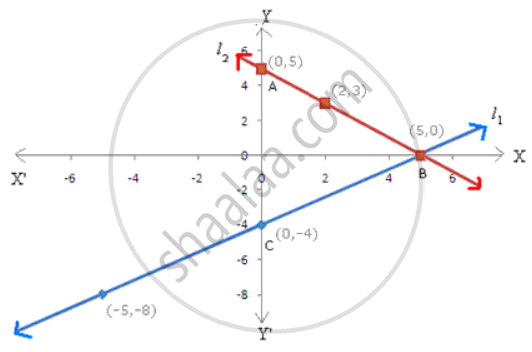
The lines l1 and l2 intersect at (5, 0). Thus, the solution of equations (1) and (2) is x = 5 and y = 0.
Now, it can be seen that ΔABC is formed by the two lines l1 and l2 and the y-axis.
The vertices of ΔABC is A(0, 5), B(5, 0) and C(0, -4).
APPEARS IN
RELATED QUESTIONS
Solve graphically the simultaneous equations given below. Take the scale as 2 cm = 1 unit on both the axes.
x - 2y - 4 = 0
2x + y = 3
The sides of a triangle are given by the equations y - 2 = 0; y + 1 = 3 (x - 2) and x + 2y = 0.
Find, graphically :
(i) the area of a triangle;
(ii) the coordinates of the vertices of the triangle.
Find graphically, the vertices of the triangle whose sides have the equations 2y - x = 8; 5y - x = 14 and y - 2x = 1 respectively. Take 1 cm = 1 unit on both the axes.
Use the graphical method to find the value of 'x' for which the expressions `(3x + 2)/(2) and (3)/(4)x - 2`
The course of an enemy submarine, as plotted on rectangular co-ordinate axes, gives the equation 2x + 3y = 4. On the same axes, a destroyer's course is indicated by the graph x - y = 7. Use the graphical method to find the point at which the paths of the submarine and the destroyer intersect?
Solve the following equations graphically :
x + 3y = 8
3x = 2 + 2y
Solve the following equations graphically :
x + 4y + 9 = 0
3y = 5x - 1
Solve the following system of equations graphically:
2x = 23 - 3y
5x = 20 + 8y
Also, find the area of the triangle formed by these lines and x-axis in each graph.
Solve graphically
3x + 2y = 4, 9x + 6y – 12 = 0
Solve graphically
y = 2x + 1, y + 3x – 6 = 0
