Advertisements
Advertisements
Question
The adjacent sides of a parallelogram are 21 cm and 28 cm. If it's one diagonal is 35 cm; find the area of the parallelogram.
Solution

First, we find the area of ΔABC.
Sides are, a = 28 cm
b = 35 cm
and c = 21 cm
S = `(a + b + c)/2`
= `(28 + 35 + 21)/2`
= `84/2 = 42` cm
area of ΔABD = `sqrt(S(S - a)(S - b)(S - c))`
= `sqrt(42(42 - 28)(42 - 35)(42 - 21))`
= `sqrt(42 xx 14 xx 7 xx 21)`
= `sqrt(2 xx 21 xx 2 xx 7 xx 7 xx 21)`
= `sqrt(2 xx 2 xx 21 xx 21 xx 7 xx 7)`
= `2 xx 21 xx 7`
= 294 cm2
∵ Diagonal of ||gm divides it into two equal parts.
∴ area of ||gm = `2 xx "area of ΔABC"`
= 2 × 294
= 588 cm2
APPEARS IN
RELATED QUESTIONS
Find the missing value:
| Base | Height | Area of parallelogram |
| 20 cm | ______ | 246 cm2 |
DL and BM are the heights on sides AB and AD respectively of parallelogram ABCD (see the given figure). If the area of the parallelogram is 1470 cm2, AB = 35 cm and AD = 49 cm, find the length of BM and DL.

The two sides of the parallelogram ABCD are 6 cm and 4 cm. The height corresponding to the base CD is 3 cm.
Find the
(i) area of the parallelogram.
(ii) the height corresponding to the base AD.

A ground is in the shape of parallelogram. The height of the parallelogram is 14 metres and the corresponding base is 8 metres longer than its height. Find the cost of levelling the ground at the rate of ₹ 15 per sq.m
What happens to the area of the parallelogram if the base is increased 2 times and the height is halved?
The height of the parallelogram is one-fourth of its base. If the area of the parallelogram is 676 sq.cm, find the height and the base
If the base and height of a parallelogram are in the ratio 7 : 3 and the height is 45 cm, then fixed the area of the parallelogram
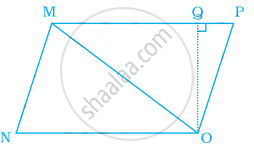
Ratio of area of ∆MNO to the area of parallelogram MNOP in the same figure is ______.
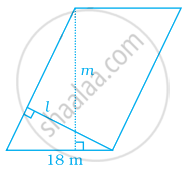
Perimeter of a parallelogram shaped land is 96 m and its area is 270 square metres. If one of the sides of this parallelogram is 18 m, find the length of the other side. Also, find the lengths of altitudes l and m in the given figure.
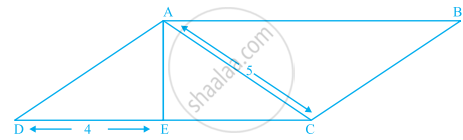
ABCD is a parallelogram in which AE is perpendicular to CD as shown in the given figure. Also, AC = 5 cm, DE = 4 cm and area of ΔAED = 6 cm2. Find the perimeter and area of parallelogram ABCD.