Advertisements
Advertisements
Question
The area of a rectangle is x2 + 7x + 12. If its breadth is (x + 3), then find its length
Solution
Let the length of the rectangle be “l”
The breadth of the rectangle = x + 3
Area of the rectangle = length × breadth
x2 + 7x + 12 = l(x + 3)
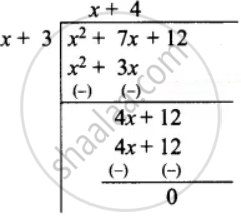
l = `(x^2 + 7x + 12)/(x + 3)`
= `((x + 4)(x + 3))/(x + 3)`
= x + 4
Length of the rectangle = x + 4
APPEARS IN
RELATED QUESTIONS
Divide the polynomial p(x) by the polynomial g(x) and find the quotient and remainder in each of the following : p(x) = x4 – 3x2 + 4x + 5, g(x) = x2 + 1 – x
Give examples of polynomials p(x), g(x), q(x) and r(x), which satisfy the division algorithm
deg r(x) = 0
If the polynomial x4 – 6x3 + 16x2 – 25x + 10 is divided by another polynomial x2 – 2x + k, the remainder comes out to be x + a, find k and a.
What must be added to the polynomial f(x) = x4 + 2x3 − 2x2 + x − 1 so that the resulting polynomial is exactly divisible by x2 + 2x − 3 ?
What must be subtracted from the polynomial f(x) = x4 + 2x3 − 13x2 − 12x + 21 so that the resulting polynomial is exactly divisible by x2 − 4x + 3 ?
Find all the zeros of the polynomial x4 + x3 − 34x2 − 4x + 120, if two of its zeros are 2 and −2.
It is given that –1 is one of the zeroes of the polynomial `x^3 + 2x^2 – 11x – 12`. Find all the zeroes of the given polynomial.
The base of a parallelogram is (5x + 4). Find its height if the area is 25x2 – 16
If on division of a polynomial p(x) by a polynomial g(x), the quotient is zero, what is the relation between the degrees of p(x) and g(x)?
Given that `x - sqrt(5)` is a factor of the cubic polynomial `x^3 - 3sqrt(5)x^2 + 13x - 3sqrt(5)`, find all the zeroes of the polynomial.
