Advertisements
Advertisements
Question
The diameters of two circular ends of the bucket are 44 cm and 24 cm . The height of the bucket is 35 cm . The capacity of the bucket is
Options
32.7 litres
33.7 litres
34.7 litres
31.7 litres
Solution
The bucket is in the form of a frustum.
The diameters are respectively, \[d_1 = 44 \text { cm and }d_2 = 24 cm\]
Radii of the circular ends = \[r_1 = 22 \text { cm and } r_2 = 12 cm\]
\[\text { Volume, V } = \frac{1}{3}\pi h\left( r_1^2 + r_1 r_2 + r_2^2 \right)\]
\[V = \frac{1}{3}\pi \times 35\left( {22}^2 + 22 \times 12 + {12}^2 \right)\]
\[V = 32706 . 6 c m^3 \]
\[ = 32 . 7\text { liters }\]
APPEARS IN
RELATED QUESTIONS
Water is flowing through a cylindrical pipe, of internal diameter 2 cm, into a cylindrical tank of base radius 40 cm, at the rate of 0.4 m/s. Determine the rise in level of water in the tank in half an hour ?
Two right circular cylinders of equal volumes have their heights in the ratio 1 : 2. What is the ratio of their radii ?
A solid metallic spherical ball of diameter 6 cm is melted and recast into a cone with diameter of the base as 12 cm. The height of the cone is
In a village, a well with 10 m inside diameter, is dug 14 m deep. Earth taken out of it is spread all around to a width 5 m to form an embankment. Find the height of the embankment. What value of the villagers is reflected here?
The radii of the circular ends of a bucket of height 15 cm are 14 cm and r cm (r < 14). If the volume of bucket is 5390 cm3, then find the value of r.
Assertion (A)
The outer surface of a hemisphere of radius 7 cm is to be painted. The total cost of painting at Rs 5 per cm2 is Rs 2300.
Reason (R)
The total surface area of a hemisphere is 3π r2.
- Both Assertion (A) and Reason (R) are true and Reason (R) is a correct explanation of Assertion (A).
- Both Assertion (A) and Reason (R) are true but Reason (R) is not a correct explanation of Assertion (A).
- Assertion (A) is true and Reason (R) is false.
- Assertion (A) is false and Reason (R) is true.
The surface area of a sphere is 616 cm2. Its radius is ______.
A cylindrical pencil sharpened at one edge is the combination of ______.
The capacity of a cylindrical vessel with a hemispherical portion raised upward at the bottom as shown in the figure is `(pir^2)/3 [3h - 2r]`.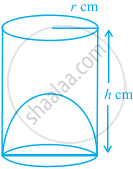
A factory manufactures 120000 pencils daily. The pencils are cylindrical in shape each of length 25 cm and circumference of base as 1.5 cm. Determine the cost of colouring the curved surfaces of the pencils manufactured in one day at Rs 0.05 per dm2.
