Advertisements
Advertisements
Question
The figure shows two identical triode tubes connected in parallel. The anodes are connected together and the cathodes are connected together. Show that the equivalent plate resistance is half the individual plate resistance, the equivalent mutual conductance is double the individual mutual conductance and the equivalent amplification factor is the same as the individual amplification factor.
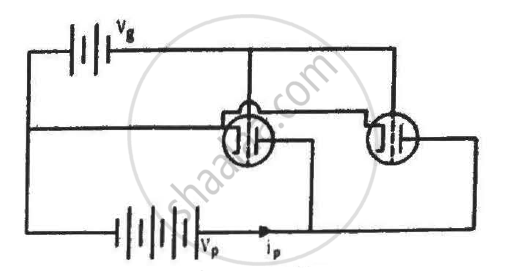
Solution
From the circuit,
Cathode of both the triodes are connected to a common point and their anodes are also connected together. Thus, the 2 triodes are connected in parallel.
As plate resistance is obtained by observing the variation of plate voltage with plate current, keeping grid voltage as constant.
Hence equivalent plate resistance
\[R_p = \frac{r_{P_1} \times r_{P_2}}{r_{P_1} + r_{P_2}}\]
if both the triodes have equal plate resistance
\[i . e . r_{P_1} = r_{P_2} = r_P \]
\[ R_P = \frac{r_P}{2}\]
To find the equivalent transconductance
We need to look at the variation of current with respect to grid voltage, keeping plate voltage as constant.
From the circuit
Now the 2 triodes are in series w.r.t grid voltage.
Hence equivalent transconductance will be equal to
\[G_m = 2 g_m \]
if the 2 triodes have equal trens conductance i.e \[g_{m_1} = g_{m_2} = g_m\]
Now the equivalent amplification factor is,
\[\mu_{\text{equivalent}} = G_m \times R_P \]
\[ \mu_{\text{equivalent}} = 2 g_m \times \frac{r_P}{2}\]
\[ \mu_{\text{equivalent}} = \mu\]
APPEARS IN
RELATED QUESTIONS
Calculate the amplification factor of a triode valve that has plate resistance of 2 kΩ and transconductance of 2 millimho.
The plate resistance and amplification factor of a triode are 10kΩ and 20. The tube is operated at plate voltage 250 V and grid voltage −7.5 V. The plate current is 10 mA. (a) To what value should the grid voltage be changed so as to increase the plate current to 15 mA? (b) To what value should the plate voltage be changed to take the plate current back to 10 mA?
The plate current, plate voltage and grid voltage of a 6F6 triode tube are related as ip = 41 (Vp + 7 Vg)1.41
Here, Vp and Vg are in volts and ip in microamperes.
The tube is operated at Vp = 250 V, Vg = −20 V. Calculate (a) the tube current, (b) the plate resistance, (c) the mutual conductance and (d) the amplification factor.
A triode has mutual conductance of 2.0 millimho and plate resistance of 20 kΩ. It is desired to amplify a signal by a factor of 30. What load resistance should be added in the circuit?
