Advertisements
Advertisements
Question
The resultant of the three concurrent space forces at A is 𝑹̅ = (-788𝒋̅) N. Find the magnitude of F1,F2 and F3 forces.

Given : A=(0,12,0)
B=(-9,0,0)
C=(0,0,5)
D=(3,0,-4)
Resultant of forces = (-788𝑗̅) N
To find : Magnitude of forces F1,F2,F3
Solution
Assume 𝑎̅,𝑏̅,𝑐̅ and 𝑑̅ be the position vectors of points A,B,C and D respectively w.r.t origin O
`bar(OA)` = 𝑎̅ = 12𝑗̅
`bar(OB)` = 𝑏̅ = -9𝑖̅
`bar(OC)`= 𝑐̅ = 5𝑘̅
`bar(OD)`= 𝑑̅ = 3𝑖̅ - 4𝑘̅
`bar(AB)`= 𝑏̅ - 𝑎̅
= -9𝑖̅-12𝑗̅
`bar(AC) `= 𝑐̅ – 𝑎̅ = 5𝑘 ̅- 12𝑗̅
`bar(AD)`= 𝑑̅ - 𝑎̅ = 3𝑖̅ -12𝑗 ̅ - 4𝑘
| Sr.no. | Vector | Magnitude |
| 1. | `bar(AB)` | 15 |
| 2. | `bar(AC) ` | 13 |
| 3. | `bar(AD)` | 13 |
| Sr.no. | Vector | `"Unit vector "= ("vector")/("Magnitude of vector")` |
| 1. | `bar(AB)` | `(−3)/5 bar(i) - 4/5 bar(j)` |
| 2. | `bar(AC) ` | `(−12)/13 bar(j) - 5/13 bar(k)` |
| 3. | `bar(AD)` | `(3)/13 bar(i) - 12/13 bar(j) - 4/13 bar(K)` |
Force along `bar(AB) = bar(F1) = F1 ((−3)/5 bar(i) - 4/5 bar(j))`
Force along `bar(AC) = bar(F2) = F2((−12)/13 bar(j) - 5/13 bar(k))`
Force along `bar(AB) = bar(F3) = F3( (3)/13 bar(i) - 12/13 bar(j) - 4/13 bar(K))`
Resultant force `bar(R) = bar(F1) +bar(F2)+ bar(F3)`
`-788 bar(j) = F1 ((−3)/5 bar(i) - 4/5 bar(j)+ bar(F2) (−12)/13 bar(j) - 5/13 bar(k) + bar(F3) (3)/13 bar(i) - 12/13 bar(j) - 4/13 bar(K))`
`0bar(j)-788 bar(j) + 0bar(k) = F1 ((−3)/5 bar(i) - 4/5 bar(j)+ bar(F2) (−12)/13 bar(j) - 5/13 bar(k) + bar(F3) (3)/13 bar(i) - 12/13 bar(j) - 4/13 bar(K))`
Comparing the equation on both sides
`(−3F1)/5 + (3F3)/13=0 `………..(1)
`-(4F1)/5--(12F2)/13 -(12F2)/13=-788` ………(2)
`(5F2)/13 -(4F3)/13 = 0 ` ……………(3)
Solving (1),(2) and (3)
F1=153.9063 N
F2=320.125 N
F3=400.1563 N
| Sr.no. | Force | Magnitude |
| 1. | F1 | 153.9063 N |
| 2. | F2 | 320.125 N |
| 3. | F3 | 400.1563 N |
APPEARS IN
RELATED QUESTIONS
Find the resultant of the force system as shown in the given figure.
In the rocket arm shown in the figure the moment of ‘F’ about ‘O’ balances that P=250 N.Find F. 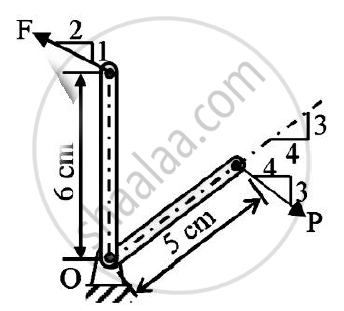
Find the force F4 , so as to give the resultant of the force as shown in the figure given below.
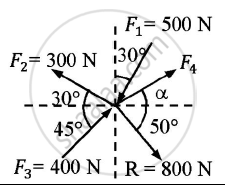
Given : Forces and their resultant
To find : Force F4
