Advertisements
Advertisements
Question
The sides of a right-angled triangle containing the right angle are 4x cm and (2x – 1) cm. If the area of the triangle is 30 cm2; calculate the lengths of its sides.
Solution
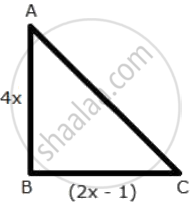
Area of triangle = 30 cm2
∴ `1/2 xx (4x) xx (2x - 1) = 30`
`2x^2 - x = 15`
`2x^2 - x - 15 = 0`
`2x^2 - 6x + 5x - 15 = 0`
`2x(x - 3) + 5(x - 3) = 0`
`(x - 3)(2x + 5) = 0`
`x = 3, (-5)/2`
But, x cannot be negative, so x = 3
Thus, we have
AB = 4 × 3 cm = 12 cm
BC = (2 × 3 – 1) cm = 5 cm
CA = `sqrt(12^2 + 5^2) cm` = 13 cm ...(Using Pythagoras theorem)
APPEARS IN
RELATED QUESTIONS
The sides of a right-angled triangle are (x – 1) cm, 3x cm and (3x + 1) cm. Find:
- the value of x,
- the lengths of its sides,
- its area.
The diagonal of a rectangle is 60 m more than its shorter side and the larger side is 30 m more than the shorter side. Find the sides of the rectangle.
A farmer has 70 m of fencing, with which he encloses three sides of a rectangular sheep pen; the fourth side being a wall. If the area of the pen is 600 sq. m, find the length of its shorter side.
The area of a big rectangular room is 300 m2. If the length were decreased by 5 m and the breadth increased by 5 m; the area would be unaltered. Find the length of the room.
A car made a run of 390 km in ‘x’ hours. If the speed had been 4 km/hour more, it would have taken 2 hours less for the journey. Find ‘x’.
The perimeter of a rectangular field is 28 m and its area is 40 sq. m. Its sides are ______.
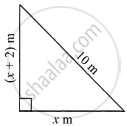
In the given figure, the value of x is ______.
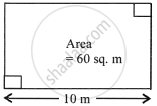
The area of the given rectangle is 60 sq. m and its longer side is 10 m, the perimeter of the rectangle is ______.
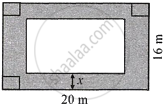
If the width of the uniform shaded portion of x m; its area in terms of x is ______.
The length of a rectangle is 3 m more than its width. If its area is 180 m2; the length of the rectangle is ______.
