Advertisements
Advertisements
Question
The specification on a heater coil is 250 V, 500 W. Calculate the resistance of the coil. What will be the resistance of a coil of 1000 W to operate at the same voltage?
Solution
Let R be the resistance of the coil.
The power P consumed by a coil of resistance R when connected across a supply V is given by
\[P = \frac{V^2}{R}\]
\[ \Rightarrow R = \frac{V^2}{P}\]
\[ \Rightarrow R = \frac{\left( 250 \right)^2}{500} = 125 \Omega\]
Now, P = 1000 W
\[\Rightarrow R = \frac{V^2}{P} = \frac{\left( 250 \right)^2}{1000} = 62 . 5 \Omega\]
APPEARS IN
RELATED QUESTIONS
Define the term 'conductivity' of a metallic wire. Write its SI unit.
Using the concept of free electrons in a conductor, derive the expression for the conductivity of a wire in terms of number density and relaxation time. Hence obtain the relation between current density and the applied electric field E.
Define current density. Write an expression which connects current density with drift speed
Define the term 'electrical conductivity' of a metallic wire. Write its S.I. unit.
The following figure shows a conductor of length l with a circular cross-section. The radius of the cross-section varies linearly from a to b. The resistivity of the material is ρ. Assuming that b – a << l, find the resistance of the conductor.

The heat developed in a system is proportional to the current through it.
A heater coil is to be constructed with a nichrome wire (ρ = 1.0 × 10−6 Ωm) that can operate at 500 W when connected to a 250 V supply. (a) What would be the resistance of the coil? (b) If the cross-sectional area of the wire is 0.5 mm2, what length of the wire will be needed? (c) If the radius of each turn is 4.0 mm, how many turns will be there in the coil?
In Figure 3 given below, find the value of resistance x for which points A and B are at the same potential:
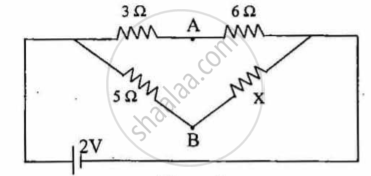
figure 3
A carbon resistor is shown in the figure. Using color code, write the value of the resistance.

Consider a current carrying wire (current I) in the shape of a circle.
Assertion: Bending a wire does not effect electrical resistance.
Reason: Resistance of wire is proportional to resistivity of material.
The electric resistance of a certain wire of iron is R. If its length and radius are both doubled, then ______.
As the temperature of conductor increase, it's resistivity and conductivity change. The ratio of resistivity to conductivity.
Consider four conducting materials copper, tungsten, mercury and aluminium with resistivity ρC, ρT, ρM and ρA respectively. Then:
