Advertisements
Advertisements
Question
The sum of first 20 odd natural numbers is
Options
100
210
400
420
Solution
Let a be the first term and d be the common difference.
We know that, sum of first n terms = Sn = \[\frac{n}{2}\][2a + (n − 1)d]
The given series is 1 + 3 + 5 + ......
First term = a = 1.
Common difference = d = 3 − 1 = 2
∴ S20 = \[\frac{20}{2}\] 2 × 1 + (20 − 1)2]
= 10(2 + 19 × 2)
= 10(40)
= 400
APPEARS IN
RELATED QUESTIONS
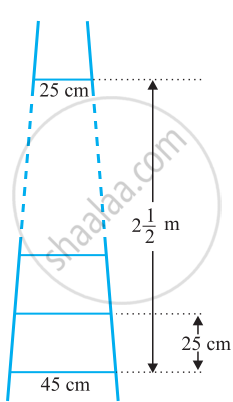
A ladder has rungs 25 cm apart. (See figure). The rungs decrease uniformly in length from 45 cm at the bottom to 25 cm at the top. If the top and bottom rungs are 2 `1/2` m apart, what is the length of the wood required for the rungs?
[Hint: number of rungs = `250/25+ 1`]
If the ratio of the sum of the first n terms of two A.Ps is (7n + 1) : (4n + 27), then find the ratio of their 9th terms.
Find the sum of the following arithmetic progressions:
1, 3, 5, 7, ... to 12 terms
How many terms are there in the A.P. whose first and fifth terms are −14 and 2 respectively and the sum of the terms is 40?
Find the sum of all natural numbers between 250 and 1000 which are divisible by 9.
The angles of quadrilateral are in whose AP common difference is 10° . Find the angles.
If the sum of first n terms is (3n2 + 5n), find its common difference.
The first and the last terms of an A.P. are 7 and 49 respectively. If sum of all its terms is 420, find its common difference.
In an AP. Sp = q, Sq = p and Sr denotes the sum of first r terms. Then, Sp+q is equal to
Find the next 4 terms of the sequence `1/6, 1/4, 1/3`. Also find Sn.
