Advertisements
Advertisements
Question
The surface area of a sphere is 2464 cm2. If its radius be doubled, then what will be the surface area of the new sphere?
Solution
Let the radii of the given sphere and the new sphere be r and R, respectively.
We have,
R = 2r and the surface area of the given sphere = 24 cm2
`i.e. 4pir^2 = 2464 cm2`
The surface area of the given sphere = 2464 cm2
`i.e 4pir^2 = 2426` cm2
The surface area of the new sphere = 4πR2
`= 4pi (2r)^2`
`= 4pi (4r)^2`
`= 4 (4pir^2)`
`= 4xx2464`
`= 9856 cm^2`
So, the surface area of the new sphere is 9856 cm2.
APPEARS IN
RELATED QUESTIONS
A well of diameter 4 m is dug 14 m deep. The earth taken out is spread evenly all around the well to form a 40 cm high embankment. Find the width of the embankment.
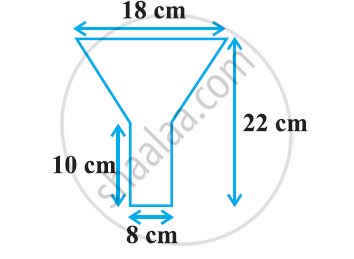
An oil funnel made of tin sheet consists of a 10 cm long cylindrical portion attached to a frustum of a cone. If the total height is 22 cm, diameter of the cylindrical portion is 8 cm and the diameter of the top of the funnel is 18 cm, find the area of the tin sheet required to make the funnel (see the given figure).
Find the surface area of a sphere of radius 7 cm.
In the middle of a rectangular field measuring 30 m × 20 m, a well of 7 m diameter and 10 m depth is dug. The earth so removed is evenly spread over the remaining part of the field. Find the height through which the level of the field is raised.
The slant height of a conical mountain is 2.5 km and the area of its base is 1.54 km2. Find the height of the mountain.
The radii of the circular ends of a bucket of height 15 cm are 14 cm and r cm (r < 14). If the volume of bucket is 5390 cm3, then find the value of r.
The volume of a cube is 729 cm3. Find its surface area.
The volume of a sphere is 4851 cm3. Find its curved surface area.
Choose the correct answer of the following question:
The radii of the circular ends of a bucket of height 40 cm are 24 cm and 15 cm. The slant height (in cm) of the bucket is
Find the number of solid spheres, each of diameter 6 cm, that could be moulded to form a solid metallic cylinder of height 45 cm and diameter 4 cm.
