Advertisements
Advertisements
Question
There is a circular path around a sports field. Sonia takes 18 minutes to drive one round of the field, while Ravi takes 12 minutes for the same. Suppose they both start at the same point and at the same time, and go in the same direction. After how many minutes will they meet again at the starting point?
Solution
Since they both move in the same direction and at the same time, to find the time when will they both meet together again, it is enough if we find the LCM of 12 and 18
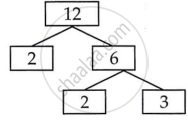 |
 |
12 = 2 × 2 × 3
18 = 2 × 3 × 3
LCM (18, 12) = 2 × 2 × 3 × 3 = 36
Therefore, Sonia and Ravi will meet again after 36 minutes.
APPEARS IN
RELATED QUESTIONS
Express the number as a product of its prime factor:
140
Determine the prime factorisation of each of the following positive integer:
58500
Find the LCM and HCF of the following integers by applying the prime factorisation method:
84, 90 and 120
Can two numbers have 16 as their HCF and 380 as their LCM? Give reason.
State Fundamental Theorem of Arithmetic.
If the prime factorization of a natural number n is 23 ✕ 32 ✕ 52 ✕ 7, write the number of consecutive zeros in n.
Find the least number that is divisible by the first ten natural numbers
The largest number which divides 60 and 75, leaving remainders 8 and 10 respectively, is ______.
The least number that is divisible by all the numbers from 1 to 10 (both inclusive) is ______.
Let a and b be two positive integers such that a = p3q4 and b = p2q3, where p and q are prime numbers. If HCF (a, b) = pmqn and LCM (a, b) = prqs, then (m + n)(r + s) = ______.
Statement A (Assertion): If product of two numbers is 5780 and their HCF is 17, then their LCM is 340.
Statement R (Reason): HCF is always a factor of LCM.
Find the HCF and LCM of 26, 65 and 117, using prime factorisation.
Two numbers are in the ratio 2 : 3 and their LCM is 180. What is the HCF of these numbers?
(HCF × LCM) for the numbers 70 and 40 is ______.
Read the following passage:
|
Khushi wants to organize her birthday party. Being health conscious, she decided to serve only fruits in her birthday party. She bought 39 apples and 60 bananas and decided to distribute fruits equally among all. |
Based on the above information, answer the following questions:
- How many guests Khushi can invite at the most?
- How many apples and bananas will each guest get?
-
- If Khushi decides to add 42 mangoes, how many guests Khushi can invite at the most?
OR - If the cost of 1 dozen of bananas is ₹ 60, the cost of 1 apple is ₹ 15 and cost of 1 mango is ₹ 20, find the total amount spent on 60 bananas, 36 apples and 42 mangoes.

- If Khushi decides to add 42 mangoes, how many guests Khushi can invite at the most?
If n is a natural number, then 8n cannot end with digit
The mean of first ten natural numbers is ______.
The HCF of two numbers 65 and 104 is 13. If LCM of 65 and 104 is 40x, then the value of x is ______.
