Advertisements
Advertisements
Question
Three charges –q, +Q, and –q are placed at equal distances on a straight line. If the potential energy of the system of the three charges is zero, then what is the ratio of Q: q?
Solution
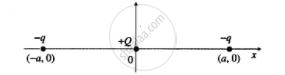
In the above figure, The x-axis is illustrated as a line joining the charges, with the origin at the +Q charge. Let q1 = +Q and q2 = q3 = –q. Since the charges are supposed to be equidistant, place the two –q charges at (–a, O) and (a, 0).
∴ r21 = r31 = a and r32 = 2a
The total potential energy of the system of three charges is
u3 = `1/(4piε_0) (("q"_1"q"_2)/"r"_21 + ("q"_1"q"_3)/"r"_31 + ("q"_2"q"_3)/"r"_32)`
`= 1/(4piε_0) [(-"q"("Q"))/"a" + ("Q"(-"q"))/"a" + ((-"q")(-"q"))/"2a"]`
`= 1/(4piε_0) [- (2"qQ")/"a" + "q"^2/"2a"]`
Given: u3 = 0
∴ `(2"qQ")/"a" = "q"^2/"2a"`
∴ `"Q"/"q" = 1/4`
This gives the required ratio.
APPEARS IN
RELATED QUESTIONS
Answer in brief:
A charge q is moved from a point A above a dipole of dipole moment p to a point B below the dipole in an equatorial plane without acceleration. Find the work done in this process.
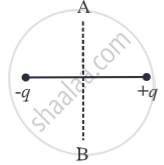
A dipole with its charges, - q and + q located at the points (O, -b, O) and (O, +b, O) is present in a uniform electric field E. The equipotential surfaces of this field are planes parallel to the yz plane.
(a) What is the direction of the electric field E?
(b) How much torque would the dipole experience in this field?
Electric potential due to the system of charges is calculated using the principle of ______
Derive an expression for the electric potential at a point due to a short electric dipole. Hence, write the expression for the electric potential at a point (i) dipole axis and (ii) on the dipole equator.
The work done in bringing a unit positive charge from infinity to a given point against the direction of electric field is known as ______.
