Question

Three concentric metallic shells A, B and C or radii a, b and c (a < b < c) have surface charge densities + σ,  −σ and + σ, respectively as shown in the figure

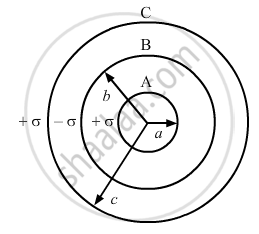
If shells A and C are at the same potential, then obtain the relation between the radii a, b and c.

Answer 1

If q_A, q_B and q_C are the charges of the respective shells, then we have:

\[q_A = 4\pi a^2 \sigma\]

\[ q_B = - 4\pi b^2 \sigma\]

\[ q_C = 4\pi c^2 \sigma\]

Let V_A and V_C be the potentials of shells A and C.
A point on the surface of shell A lies inside the shells B and C.

\[\therefore V_A = \frac{1}{4 \pi\epsilon_0} . \frac{q_A}{a} + \frac{1}{4 \pi\epsilon_0} . \frac{q_B}{b} + \frac{1}{4 \pi\epsilon_0} . \frac{q_C}{c}\]

\[ = \frac{1}{4 \pi\epsilon_0}\left( \frac{4\pi a^2 \sigma}{a} - \frac{4\pi b^2 \sigma}{b} + \frac{4\pi c^2 \sigma}{c} \right)\]

\[ \Rightarrow V_A = \frac{\sigma}{\epsilon_0}\left( a - b + c \right)\]

A point on shell C lies outside both A and B.

\[\therefore V_C = \frac{1}{4 \pi\epsilon_0}\left( \frac{q_A}{c} + \frac{q_B}{c} + \frac{q_C}{c} \right)\]

\[ = \frac{1}{4 \pi\epsilon_0}\left( \frac{4\pi a^2 \sigma}{c} - \frac{4\pi b^2 \sigma}{c} + \frac{4\pi c^2 \sigma}{c} \right)\]

\[ \Rightarrow V_C = \frac{\sigma}{\epsilon_0}\left( \frac{a^2 - b^2}{c} + c \right)\]

If the shells A and C are at the same potential, then V_A = V_C.

\[i . e . , \frac{\sigma}{\epsilon_0}\left( a - b + c \right) = \frac{\sigma}{\epsilon_0}\left( \frac{a^2 - b^2}{c} + c \right)\]

\[ \Rightarrow \left( a - b + c \right) = \frac{a^2 - b^2}{c} + c\]

\[ \Rightarrow c\left( a - b \right) = a^2 - b^2 \]

\[ \Rightarrow c = a + b\]