Question

Two point charges of magnitude +q and –q are placed at (–d/2, 0, 0) and (d/2, 0, 0), respectively. Find the equation of the equipotential surface where the potential is zero.

Answer 1

Let the plane be at a distance x from the origin. The potential at the point P is

`1/(4piε_0) q/([(x + d/2)^2 + h^2]^(1/2)) - 1/(4piε_0) q/([(x - d/2)^2 + h^2]^(1/2))`

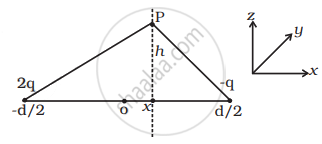
If this is to be zero.

`1/([(x + d/2)^2 + h^2]^(1/2)) = 1/([(x - d/2)^2 + h^2]^(1/2))`

Or, (x - d/2)² + h² = (x + d/2)² + h² 

⇒ x² – dx + d² /4 = x² + dx + d² /4

Or, 2dx = 0

⇒ x = 0

The equation is that of a plane x = 0.