Advertisements
Advertisements
Question
Using Euler's formula, find the values of x, y, z.
| Faces | Vertices | Edges | |
| (i) | x | 15 | 20 |
| (ii) | 6 | y | 8 |
| (iii) | 14 | 26 | z |
Solution
We will solve for x, y, and z using Euler's formula for polyhedra:
V − E + F = 2
Where:
- V = Number of vertices,
- E = Number of edges,
- F = Number of faces.
(i) Find x (Number of Faces)
- V = 15,
- E = 20
- F = x
Using Euler's formula:
15 − 20 + x = 2
−5 + x = 2
x = 7
(ii) Find y (Number of Vertices)
- V = y,
- E = 8
- F = 6
Using Euler's formula:
y − 8 + 6 = 2
y − 2 = 2
y = 4
(iii) Find z (Number of Edges)
Given:
- V = 26,
- E = z
- F = 14
26 − z + 14 = 2
40 − z = 2
z = 38
Final Answers:
- x = 7,
- y = 4
- z = 38
APPEARS IN
RELATED QUESTIONS
Verify Euler’s formula for the following three-dimensional figures:

In a solid if F = V = 5, then the number of edges in this shape is ______.
Which of the following cannot be true for a polyhedron?
If a solid shape has 12 faces and 20 vertices, then the number of edges in this solid is ______.
Look at the shapes given below and state which of these are polyhedra using Euler’s formula.

Look at the shapes given below and state which of these are polyhedra using Euler’s formula.
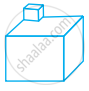
Look at the shapes given below and state which of these are polyhedra using Euler’s formula.
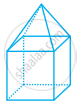
Look at the shapes given below and state which of these are polyhedra using Euler’s formula.
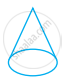
Look at the shapes given below and state which of these are polyhedra using Euler’s formula.

Using Euler’s formula, find the value of unknown r in the following table.
| Faces | 8 |
| Vertices | 11 |
| Edges | r |
