Topics
Similarity
- Similarity of Triangles
- Properties of Ratios of Areas of Two Triangles
- Basic Proportionality Theorem (Thales Theorem)
- Converse of Basic Proportionality Theorem
- Property of an Angle Bisector of a Triangle
- Property of Three Parallel Lines and Their Transversals
- Similar Triangles
- Criteria for Similarity of Triangles
- Areas of Similar Triangles
Pythagoras Theorem
- Pythagoras Theorem
- Pythagorean Triplet
- Property of 30°- 60°- 90° Triangle Theorem
- Property of 45°- 45°- 90° Triangle Theorem
- Similarity in Right Angled Triangles
- Theorem of Geometric Mean
- Right-angled Triangles and Pythagoras Property
- Converse of Pythagoras Theorem
- Application of Pythagoras Theorem in Acute Angle and Obtuse Angle
- Apollonius Theorem
Circle
- Concept of Circle
- Circles Passing Through One, Two, Three Points
- Secant and Tangent
- Tangent to a Circle
- Converse of Tangent Theorem
- Tangent Segment Theorem
- Touching Circles
- Theorem of Touching Circles
- Tangent Properties - If Two Circles Touch, the Point of Contact Lies on the Straight Line Joining Their Centers
- Introduction to an Arc
- Congruence of Arcs
- Property of Sum of Measures of Arcs
- Inscribed Angle
- Intercepted Arc
- Inscribed Angle Theorem
- Corollaries of Inscribed Angle Theorem
- Cyclic Quadrilateral
- Theorem: Opposite angles of a cyclic quadrilateral are supplementary.
- Corollary of Cyclic Quadrilateral Theorem
- Converse: If a Pair of Opposite Angles of a Quadrilateral is Supplementary, Then the Quadrilateral is Cyclic.
- Converse of Cyclic Quadrilateral Theorem
- Theorem of Angle Between Tangent and Secant
- Converse of Theorem of the Angle Between Tangent and Secant
- Theorem of Internal Division of Chords
- Theorem of External Division of Chords
- Tangent Secant Segments Theorem
- Tangent - Secant Theorem
- Angle Subtended by the Arc to the Point on the Circle
- Angle Subtended by the Arc to the Centre
- Number of Tangents from a Point on a Circle
Geometric Constructions
- Basic Geometric Constructions
- Division of a Line Segment
- Construction of Similar Triangle
- Construction of a Tangent to the Circle at a Point on the Circle
- To Construct Tangents to a Circle from a Point Outside the Circle.
Co-ordinate Geometry
- Coordinate Geometry
- Distance Formula
- Intercepts Made by a Line
- Division of a Line Segment
- Section Formula
- The Mid-point of a Line Segment (Mid-point Formula)
- Centroid Formula
- Slope of a Line
- General Equation of a Line
- Standard Forms of Equation of a Line
Trigonometry
Mensuration
- Conversion of Solid from One Shape to Another
- Euler's Formula
- Concept of Surface Area, Volume, and Capacity
- Surface Area and Volume of Three Dimensional Figures
- Surface Area and Volume of Different Combination of Solid Figures
- Frustum of a Cone
- Sector of a Circle
- Area of a Sector of a Circle
- Length of an Arc
- Segment of a Circle
- Area of a Segment
- Circumference of a Circle
- Areas of Sector and Segment of a Circle
Formula
Euler’s formula ⇒ F + V – E = 2.
Notes
Euler’s formula:
For any polyhedron, F + V – E = 2
Where ‘F’ stands for a number of faces, V stands for a number of vertices and E stands for a number of edges. This relationship is called Euler’s formula.
| Solid | Shape | F | V | E | F + V | E + 2 |
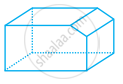
| Cuboid |  |
6 | 8 | 12 | 14 | 14 |
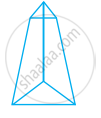
| Triangular Pyramid |  |
4 | 4 | 6 | 8 | 8 |
| Triangular Prism | 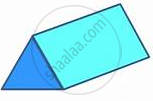 |
5 | 6 | 9 | 11 | 11 |
| Pyramid with square base | 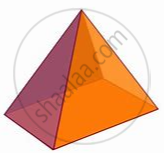 |
5 | 5 | 8 | 10 | 10 |
| Prism with square base |  |
6 | 8 | 12 | 14 | 14 |
If you would like to contribute notes or other learning material, please submit them using the button below.
Shaalaa.com | What is Euler's Formula?
to track your progress
Related QuestionsVIEW ALL [34]
Using Euler’s formula, find the value of unknown y in the following table.
| Faces | y |
| Vertices | 12 |
| Edges | 18 |
Using Euler's formula, find the values of x, y, z.
| Faces | Vertices | Edges | |
| (i) | x | 15 | 20 |
| (ii) | 6 | y | 8 |
| (iii) | 14 | 26 | z |
Complete the table given below:
| S.No | Solid | Shape of Solid |
Number of faces F |
Number of Verticles V |
Number of edges E |
F + V | E + 2 |
| a. | Cuboid |  |
|||||
| b. | Triangular Pyramid |
 |
|||||
| c. | Square Pyramid |
 |
|||||
| d. | Rectangular Pyramid |
 |
|||||
| e. | Pentagonal Pyramid |
 |
|||||
| f. | Hexagonal Pyramid |
 |
|||||
| g. | Triangular Prism |
 |
|||||
| h. | Square Prism |
 |
|||||
| i. | Cube |  |
|||||
| j. | Pentagonal Prism |
 |
|||||
| k. | Octagonal Prism |
 |
|||||
| l. | Heptagonal Prism |
 |