Topics
Similarity
- Similarity of Triangles
- Properties of Ratios of Areas of Two Triangles
- Basic Proportionality Theorem (Thales Theorem)
- Converse of Basic Proportionality Theorem
- Property of an Angle Bisector of a Triangle
- Property of Three Parallel Lines and Their Transversals
- Similar Triangles
- Criteria for Similarity of Triangles
- Areas of Similar Triangles
Pythagoras Theorem
- Pythagoras Theorem
- Pythagorean Triplet
- Property of 30°- 60°- 90° Triangle Theorem
- Property of 45°- 45°- 90° Triangle Theorem
- Similarity in Right Angled Triangles
- Theorem of Geometric Mean
- Right-angled Triangles and Pythagoras Property
- Converse of Pythagoras Theorem
- Application of Pythagoras Theorem in Acute Angle and Obtuse Angle
- Apollonius Theorem
Circle
- Concept of Circle
- Circles Passing Through One, Two, Three Points
- Secant and Tangent
- Tangent to a Circle
- Converse of Tangent Theorem
- Tangent Segment Theorem
- Touching Circles
- Theorem of Touching Circles
- Tangent Properties - If Two Circles Touch, the Point of Contact Lies on the Straight Line Joining Their Centers
- Introduction to an Arc
- Congruence of Arcs
- Property of Sum of Measures of Arcs
- Inscribed Angle
- Intercepted Arc
- Inscribed Angle Theorem
- Corollaries of Inscribed Angle Theorem
- Cyclic Quadrilateral
- Theorem: Opposite angles of a cyclic quadrilateral are supplementary.
- Corollary of Cyclic Quadrilateral Theorem
- Converse: If a Pair of Opposite Angles of a Quadrilateral is Supplementary, Then the Quadrilateral is Cyclic.
- Converse of Cyclic Quadrilateral Theorem
- Theorem of Angle Between Tangent and Secant
- Converse of Theorem of the Angle Between Tangent and Secant
- Theorem of Internal Division of Chords
- Theorem of External Division of Chords
- Tangent Secant Segments Theorem
- Tangent - Secant Theorem
- Angle Subtended by the Arc to the Point on the Circle
- Angle Subtended by the Arc to the Centre
- Number of Tangents from a Point on a Circle
Geometric Constructions
- Basic Geometric Constructions
- Division of a Line Segment
- Construction of Similar Triangle
- Construction of a Tangent to the Circle at a Point on the Circle
- To Construct Tangents to a Circle from a Point Outside the Circle.
Co-ordinate Geometry
- Coordinate Geometry
- Distance Formula
- Intercepts Made by a Line
- Division of a Line Segment
- Section Formula
- The Mid-point of a Line Segment (Mid-point Formula)
- Centroid Formula
- Slope of a Line
- General Equation of a Line
- Standard Forms of Equation of a Line
Trigonometry
Mensuration
- Conversion of Solid from One Shape to Another
- Euler's Formula
- Concept of Surface Area, Volume, and Capacity
- Surface Area and Volume of Three Dimensional Figures
- Surface Area and Volume of Different Combination of Solid Figures
- Frustum of a Cone
- Sector of a Circle
- Area of a Sector of a Circle
- Length of an Arc
- Segment of a Circle
- Area of a Segment
- Circumference of a Circle
- Areas of Sector and Segment of a Circle
Notes
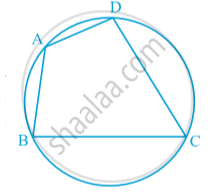
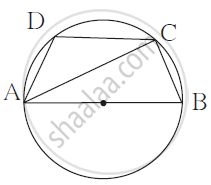
A quadrilateral ABCD is called cyclic if all the four vertices of it lie on a circle in fig. A quadrilateral is said to be a cyclic quadrilateral, if there is a circle passing through all its four vertices.
`=>`∠A + ∠C = 180°
also ∠B + ∠D = 180°.
Theorem
Theorem: The sum of either pair of opposite angles of a cyclic quadrilateral is 180º.
Given : A cyclic quadrilateral ABCD .
To Prove : ∠BAD + ∠BCD = ∠ABC + ∠ADC = 180º.
Construction : Draw AC and DB.
Proof : ∠ACB = ∠ ADB and
∠BAC = ∠BDC [Angles in the same segment]
`therefore` ∠ACB + ∠BAC = ∠ADB +∠BDC = ∠ADC
Adding ∠ABC on both the sides, we get
∠ACB + ∠BAC +∠ABC = ∠ADC +∠ABC
But ∠ACB +∠BAC +∠ABC = 180º. [sum of the angles of a triangle]
`therefore` ∠ ADC + ∠ABC =180º.
`therefore` ∠BAD +∠BCD = 360º - (∠ADC + ∠ABC) = 180º.
Hence proved.
Theorem: If the sum of a pair of opposite angles of a quadrilateral is 180º, the quadrilateral is cyclic.
Video Tutorials
Shaalaa.com | Circles Theorem: Cyclic quadrilateral
Series: 1
00:02:37 undefined
00:10:11 undefined
00:07:49 undefined
00:09:06 undefined
00:10:23 undefined
00:07:37 undefined
00:08:07 undefined
00:07:59 undefined
00:10:14 undefined