Question

Will a line pass through (2, 2) if it intersects the axes at (2, 0) and (0, 2)

Answer 1

Given a line intersects the axis at (2, 0) & (0, 2)

Let line intercept form be expressed as

ax + by = 1 Where a & b are the x & y intercept respectively.

Since the intercept points are (2, 0) & (0, 2)

a = 2, b = 2

∴ 2x + 2y = 1

When the point (2, 2) is considered & substituted in the equation

2x + 2y = 1, we get

2 × 2 + 2 × 2 = 4 ≠ 1

∴ the point (2, 2) does not satisfy the equation.

Therefore the line does not pass through (2, 2)

Alternatively graphical method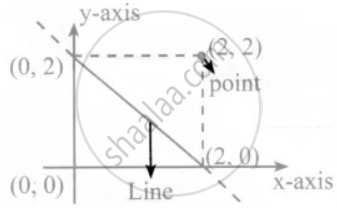

as we can see the line doesn’t pass through (2, 2)