Advertisements
Chapters
![Samacheer Kalvi solutions for Mathematics [English] Class 8 TN Board chapter 3 - Algebra Samacheer Kalvi solutions for Mathematics [English] Class 8 TN Board chapter 3 - Algebra - Shaalaa.com](/images/mathematics-english-class-8-tn-board_6:5f2b1b2038084cf381bfa42c826a928c.jpg)
Advertisements
Solutions for Chapter 3: Algebra
Below listed, you can find solutions for Chapter 3 of Tamil Nadu Board of Secondary Education Samacheer Kalvi for Mathematics [English] Class 8 TN Board.
Samacheer Kalvi solutions for Mathematics [English] Class 8 TN Board 3 Algebra Exercise 3.1 [Pages 81 - 82]
Complete the table.
| × | 2x2 | −2xy | x4y3 | 2xyz | (___)xz2 |
| x4 | |||||
| (___) | 4x5y4 | ||||
| −x2y | |||||
| 2y2z | −10xy2z3 | ||||
| −3xyz | |||||
| (___) | −14xyz2 |
Find the product of the terms
−2mn, (2m)2, −3mn
Find the product of the terms
3x2y , −3xy3, x2y2
If l = 4pq2, b = −3p2q, h = 2p3q3 then, find the value of l × b × h
Expand 5x(2y – 3)
Expand −2p(5p2 – 3p + 7)
Expand 3mn(m3n3 – 5m2n + 7mn2)
Expand x2(x + y + z) + y2(x + y + z) + z2(x – y – z)
Find the product of (2x + 3)(2x – 4)
Find the product of (y2 – 4)(2y2 + 3y)
Find the product of (m2 – n)(5m2n2 – n2)
Find the product of 3(x – 5) × 2(x – 1)
Find the missing term
6xy × _________ = –12x3y
Find the missing term
_________ × (−15m2n3p) = 45m3n3p2
Find the missing term
2y(5x2y – ___ + 3 ___ ) = 10x2y2 – 2xy + 6y3
Match the following
Match the following:
| Column A | Column B |
| (a) 4y2 × −3y | (i) 20x2y −20x |
| (b) −2xy(5x2 −3) | (ii) 5x3 − 5xy2 + 5x2y |
| (c) 5x(x2 − y2 + xy) | (iii) 4x2 − 9 |
| (d) (2x + 3)(2x −3) | (iv) −12y3 |
| (e) 5x(4xy −4) | (v) −10x3y + 6xy |
iv, v, ii, i, iii
v, iv, iii, ii, i
iv, v, ii, iii, i
iv, v, iii, ii, i
A car moves at a uniform speed of (x + 30) km/hr. Find the distance covered by the car in (y + 2)hours. (Hint: distance = speed × time).
Objective Type Questions
The product of 7p3 and (2p2)2 is
14p12
28p7
9p7
11p12
The missing terms in the product −3m3n × 9(__) = _________ m4n3
mn2, 27
m2n, 27
m2n2, – 27
mn2, – 27
If the area of a square is 36x4y2 then, its side is ____________
6x4y2
8x2y2
6x2y
−6x2y
If the area of a rectangle is 48m2n3 and whose length is 8mn2 then, its breadth is _______
6 mn
8m2n
7m2n2
6m2n2
If the area of a rectangular land is (a2 – b2) sq.units whose breadth is (a – b) then, its length is __________
a – b
a + b
a2 – b
(a + b)2
Samacheer Kalvi solutions for Mathematics [English] Class 8 TN Board 3 Algebra Exercise 3.2 [Pages 85 - 86]
Fill in the blanks:
`(18"m"^4(_))/(2"m"^3"n"^3` = _____ mn5
`("l"^4"m"^5"n"^((_)))/(2"l""m"^((_))"n"^6) = ("l"^3"m"^3"n")/((_))`
`(42"a"^4"b"^5(_))/(6"a"^4"b"^2)` = `(_)"b"^((_))"c"^2`
Say True or False
8x3y ÷ 4x2 = 2xy
True
False
7ab3 ÷ 14ab = 2b2
True
False
Divide 27y3 by 3y
Divide x3y2 by x2y
Divide 45x3y2z4 by (−15xyz)
Divide (3xy)2 by 9xy
Simplify `(3"m"^2)/"m" + (2"m"^4)/("m"^3)`
Simplify `(14"p"^5"q"^3)/(2"p"^2"q") - (12"p"^3"q"^4)/(3"q"^2)`
Divide: (32y2 – 8yz) by 2y
Divide: (4m2n3 + 16m4n2 – mn) by 2mn
Divide:
5xy2 – 18x2y3 + 6xy by 6xy
Divide: 81(p4q2r3 + 2p3q3r2 – 5p2q2r2) by (3pqr)2
Identify the errors and correct them
7y2 – y2 + 3y2 = 10y2
Identify the errors and correct them
6xy + 3xy = 9x2y2
Identify the errors and correct them
m(4m – 3) = 4m2 – 3
Identify the errors and correct them
(4n)2 – 2n + 3 = 4n2 – 2n + 3
Identify the errors and correct them
(x – 2)(x + 3) = x2 – 6
Identify the errors and correct them
– 3p2 + 4p – 7 = – (3p2 + 4p – 7)
Statement A: If 24p2q is divided by 3pq, then the quotient is 8p.
Statement B: Simplification of `((5x + 5))/5` is 5x
Both A and B are true
A is true but B is false
A is false but B is true
Both A and B are false
Statement A: 4x2 + 3x – 2 = `2(2x^2 + (3x)/2 - 1)`
Statement B: (2m – 5) – (5 – 2m) = (2m – 5) + (2m – 5)
Both A and B are true
A is true but B is false
A is false but B is true
Both A and B are false
Samacheer Kalvi solutions for Mathematics [English] Class 8 TN Board 3 Algebra Exercise 3.3 [Pages 91 - 92]
Expand (3m + 5)2
Expand (5p – 1)2
Expand (2n – 1)(2n + 3)
Expand 4p2 – 25q2
Expand (3 + m)3
Expand (2a + 5)3
Expand (3p + 4q)3
Expand (52)3
Expand (104)3
Expand (5 − x)3
Expand (2x – 4y)3
Expand (ab – c)3
Expand (48)3
Expand (97xy)3
Simplify (p – 2)(p + 1)(p – 4)
Find the volume of the cube whose side is (x + 1) cm
Find the volume of the cuboid whose dimensions are (x + 2), (x – 1) and (x – 3)
Objective Type Questions
If x2 – y2 = 16 and (x + y) = 8 then (x – y) is ___________
8
3
2
1
`(("a" + "b")("a"^3 - "b"^3))/(("a"^2 - "b"^2))` = ___________
a2 – ab + b2
a2 + ab + b2
a2 + 2ab + b2
a2 – 2ab + b2
(p + q)(p2 – pq + q2) is equal to _____________
p3 + q3
(p + q)3
p3 – q3
(p – q)3
(a – b) = 3 and ab = 5 then a3 – b3 = __________
15
18
62
72
a3 + b3 = (a + b)3 = __________
3a(a + b)
3ab(a – b)
– 3ab(a + b)
3ab(a + b)
Samacheer Kalvi solutions for Mathematics [English] Class 8 TN Board 3 Algebra Exercise 3.4 [Page 96]
Factorise the following by taking out the common factor
18xy – 12yz
Factorise the following by taking out the common factor
9x5y3 + 6x3y2 – 18x2y
Factorise the following by taking out the common factor
x(b – 2c) + y(b – 2c)
Factorise the following by taking out the common factor
(ax + ay) + (bx + by)
Factorise the following by taking out the common factor
2x2(4x – 1) – 4x + 1
Factorise the following by taking out the common factor
3y(x – 2)2 – 2(2 – x)
Factorise the following by taking out the common factor
6xy – 4y2 + 12xy – 2yzx
Factorise the following by taking out the common factor
a3 – 3a2 + a – 3
Factorise the following by taking out the common factor
3y3 – 48y
Factorise the following by taking out the common factor
ab2 – bc2 – ab + c2
Factorise the following expressions
x2 + 14x + 49
Factorise the following expressions
y2 – 10y + 25
Factorise the following expressions
c2 – 4c – 12
Factorise the following expressions
m2 + m – 72
Factorise the following expressions
4x2 – 8x + 3
Factorise the following expressions using (a + b)3 = a3 + 3a2b + 3ab2 + b3 identity
64x3 + 144x2 + 108x + 27
Factorise the following expressions using (a + b)3 = a3 + 3a2b + 3ab2 + b3 identity
27p3 + 54p2q + 36pq2 + 8q3
Factorise the following expressions using (a – b)3 = a3 – 3a2b + 3ab2 – b3 identity
y3 – 18y2 + 108y – 216
Factorise the following expressions using (a – b)3 = a3 – 3a2b + 3ab2 – b3 identity
8m3 – 60m2n + 150mn2 – 125n3
Objective type Questions
Factors of 9x2 + 6xy are
3y, (x + 2)
3x, (3x + 3y)
6x, (3x + 2y)
3x, (3x + 2y)
Factors of 4 – m2 are
(2 + m)(2 + m)
(2 – m)(2 – m)
(2 + m)(2 – m)
(4 + m)(4 – m)
(x + 4) and (x – 5) are the factors of ___________
x2 – x + 20
x2 – 9x – 20
x2 + x – 20
x2 – x – 20
The factors of x2 – 5x + 6 are (x – 2)(x – p) then the value of p is ______.
– 3
3
2
– 2
The factors of 1 – m3
(1 + m), (1 + m + m2)
(1 – m), (1 – m – m2)
(1 – m), (1 + m + m2)
(1 + m), (1 – m + m2)
One factor of x3 + y3 is
(x – y)
(x + y)
(x + y)3
(x – y)3
Samacheer Kalvi solutions for Mathematics [English] Class 8 TN Board 3 Algebra Exercise 3.5 [Page 97]
Miscellaneous Practice Problems
Subtract: −2(xy)2 (y3 + 7x2y + 5) from 5y2 (x2y3 – 2x4y + 10x2)
Multiply (4x2 + 9) and (3x – 2)
Find the simple interest on Rs. 5a2b2 for 4ab years at 7b% per annum
The cost of a notebook is Rs. 10ab. If Babu has Rs. (5a2b + 20ab2 + 40ab). Then how many notebooks can he buy?
Factorise: (7y2 – 19y – 6)
Challenging problems
A contractor uses the expression 4x2 + 11x + 6 to determine the amount of wire to order when wiring a house. If the expression comes from multiplying the number of rooms times the number of outlets and he knows the number of rooms to be (x + 2), find the number of outlets in terms of ’x’. [Hint : factorise 4x2 + 11x + 6]
A mason uses the expression x2 + 6x + 8 to represent the area of the floor of a room. If the decides that the length of the room will be represented by (x + 4), what will the width of the room be in terms of x?
Find the missing term: y2 + (...)x + 56 = (y + 7)(y + ...)
Factorise : 16p4 – 1
Factorise : 3x3 – 45x2y + 225xy2 – 375y3
Samacheer Kalvi solutions for Mathematics [English] Class 8 TN Board 3 Algebra Exercise 3.6 [Page 102]
Fill in the blanks:
The value of x in the equation x + 5 = 12 is _________
The value of y in the equation y – 9 = (– 5) + 7 is ________
The value of m in the equation 8m = 56 is ________
The value of p in the equation `(2"p")/3` = 10 is ________
The linear equation in one variable has ________ solution
Say True or False
The shifting of a number from one side of an equation to other is called transposition
True
False
Linear equation in one variable has only one variable with power 2
True
False
Match the following :
| Column A | Column B |
| (a) `x/2` = 10 | (i) x = 4 |
| (b) 20 = 6x − 4 | (ii) x = 1 |
| (c) 2x − 5 = 3 − x | (iii) x = 20 |
| (d) 7x − 4 − 8x = 20 | (iv) x = `8/3` |
| (e) `4/11 - x = (-7)/11` | (v) x = −24 |
(i), (ii), (iv), (iii), (v)
(iii), (iv), (i), (ii), (v)
(iii), (i), (iv), (v), (ii)
(iii), (i), (v), (iv), (ii)
Find x and p: `(2x)/3 - 4 = 10/3`
Find x and p: `y + 1/6 - 3y = 2/3`
Find x and p: `1/3 - x/3 = (7x)/12 + 5/4`
Find x: −3(4x + 9) = 21
Find x: 20 – 2(5 – p) = 8
Find x: (7x – 5) – 4(2 + 5x) = 10(2 – x)
Find x and m: `(3x - 2)/4 - ((x - 3))/5` = −1
Find x and m: `("m" + 9)/(3"m" + 15) = 5/3`
Samacheer Kalvi solutions for Mathematics [English] Class 8 TN Board 3 Algebra Exercise 3.7 [Pages 106 - 107]
Fill in the blanks:
The solution of the equation ax + b = 0 is _______
If a and b are positive integers then the solution of the equation ax = b has to be always _______
One-sixth of a number when subtracted from the number itself gives 25. The number is _______
If the angles of a triangle are in the ratio 2 : 3 : 4 then the difference between the greatest and the smallest angle is _______
In an equation a + b = 23. The value of a is 14 then the value of b is _______
Say True or False
“Sum of a number and two times that number is 48” can be written as y + 2y = 48
True
False
5(3x + 2) = 3(5x – 7) is a linear equation in one variable
True
False
x = 25 is the solution of one third of a number is less than 10 the original number.
True
False
One number is seven times another. If their difference is 18, find the numbers
The sum of three consecutive odd numbers is 75. Which is the largest among them?
The length of a rectangle is `1/3` of its breadth. If its perimeter is 64 m, then find the length and breadth of the rectangle.
A total of 90 currency notes, consisting only of ₹ 5 and ₹ 10 denominations, amount to ₹ 500. Find the number of notes in each denomination.
At present, Thenmozhi’s age is 5 years more than that of Murali’s age. Five years ago, the ratio of Thenmozhi’s age to Murali’s age was 3 : 2. Find their present ages.
A number consists of two digits whose sum is 9. If 27 is subtracted from the original number, its digits are interchanged. Find the original number
The denominator of a fraction exceeds Its numerator by 8. If the numerator is increased by 17 and the denominator is decreased by 1, we get `3/2`. Find the original fraction.
If a train runs at 60 km/hr it reaches its destination late by 15 minutes. But, if it runs at 85 kmph it is late by only 4 minutes. Find the distance to be covered by the train.
Objective Type Questions
Sum of a number and its half is 30 then the number is ______
15
20
25
40
The exterior angle of a triangle is 120° and one of its interior opposite angle 58°, then the other opposite interior angle is _________
62°
72°
78°
68°
What sum of money will earn ₹ 500 as simple interest in 1 year at 5% per annum?
50000
30000
10000
5000
The product of L.C.M and H.C.F of two numbers is 24. If one of the number is 6, then the other number is ________
6
2
4
8
The largest number of the three consecutive numbers is x + 1, then the smallest number is ________
x
x + 1
x + 2
x – 1
Samacheer Kalvi solutions for Mathematics [English] Class 8 TN Board 3 Algebra Exercise 3.8 [Pages 111 - 112]
Fill in the blanks:
X-axis and Y-axis intersect at _________
The coordinates of the point in third quadrant are always _________
(0, −5) point lies on _________ axis
The x-coordinate is always ______ on the y-axis
___________ coordinates are the same for a line parallel to Y-axis
Say True or False:
(−10, 20) lies in the second quadrant
True
False
(−9, 0) lies on the x-axis
True
False
The coordinates of the origin are (1, 1)
True
False
Find the quadrants without plotting the point on a graph sheet
(3, −4)
Find the quadrants without plotting the point on a graph sheet.
(5, 7)
Find the quadrants without plotting the point on a graph sheet.
(2, 0)
Find the quadrants without plotting the point on a graph sheet
(−3, −5)
Find the quadrants without plotting the point on a graph sheet
(4, −3)
Find the quadrants without plotting the point on a graph sheet
(−7, 2)
Find the quadrants without plotting the point on a graph sheet.
(−8, 0)
Find the quadrants without plotting the point on a graph sheet.
(0, 10)
Find the quadrants without plotting the point on a graph sheet.
(−9, 50)
Plot the following point in a graph sheet.
A(5, 2)
Plot the following point in a graph sheet.
B(−7, −3)
Plot the following point in a graph sheet.
C(−2, 4)
Plot the following point in a graph sheet.
D(−1, −1)
Plot the following point in a graph sheet.
E(0, −5)
Plot the following point in a graph sheet.
F(2, 0)
Plot the following point in a graph sheet.
G(7, −4)
Plot the following point in a graph sheet.
H(−4, 0)
Plot the following point in a graph sheet.
I(2, 3)
Plot the following point in a graph sheet.
J(8, −4)
Plot the following point in a graph sheet.
K(0, 7)
Use the graph to determine the coordinates where each figure is located.
| a) Star | _______ |
| b) Bird | _______ |
| c) Red circle | _______ |
| d) Diamond | _______ |
| e) Triangle | _______ |
| f) Ant | _______ |
| g) Mango | _______ |
| h) Housefly | _______ |
| i) Medal | _______ |
| j) Spider | _______ |
Samacheer Kalvi solutions for Mathematics [English] Class 8 TN Board 3 Algebra Exercise 3.9 [Pages 117 - 118]
Fill in the blanks:
y = px where p ∈ Z always passes through the _________
The intersecting point of the line x = 4 and y = −4 is _________
Scale for the given graph,
On the x-axis 1 cm = _________ units
y-axis 1 cm = _________ units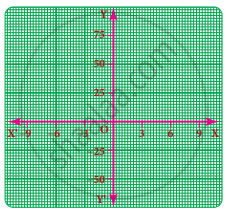
Say True or False:
The points (1, 1) (2, 2) (3, 3) lie on a same straight line
True
False
y = −9x not passes through the origin
True
False
Will a line pass through (2, 2) if it intersects the axes at (2, 0) and (0, 2)
A line passing through (4, – 2) and intersects the Y-axis at (0, 2). Find a point on the line in the second quadrant
If the points P(5, 3) Q(−3, 3) R(−3, −4) and S form a rectangle then find the coordinate of S
A line passes through (6, 0) and (0, 6) and an another line passes through (−3, 0) and (0, −3). What are the points to be joined to get a trapezium?
Find the point of intersection of the line joining points (– 3, 7) (2, – 4) and (4, 6) (–5, – 7). Also, find the point of intersection of these lines and also their intersection with the axis.
Draw the graph of the following equation:
x = – 7
Draw the graph of the following equation:
y = 6
Draw the graph of y = – 3x
Draw the graph of y = x – 4
Draw the graph of y = 2x + 5
Find the values.
y = x + 3
| x | 0 | − 2 | ||
| y | 0 | − 3 |
Find the values.
2x + y − 6 = 0
| x | 0 | − 1 | ||
| y | 0 | − 2 |
Find the values.
y = 3x + 1
| x | − 1 | 0 | 1 | 2 |
| y |
Samacheer Kalvi solutions for Mathematics [English] Class 8 TN Board 3 Algebra Exercise 3.10 [Pages 118 - 119]
Miscellaneous Practice Problems
The sum of three numbers is 58. The second number is three times of two-fifth of the first number and the third number is 6 less than the first number. Find the three numbers
In triangle ABC, the measure of ∠B is two-third of the measure of ∠A. The measure of ∠C is 20° more than the measure of ∠A. Find the measures of the three angles.
Two equal sides of an isosceles triangle are 5y – 2 and 4y + 9 units. The third side is 2y + 5 units. Find ‘y’ and the perimeter of the triangle.
In the given figure, angle XOZ and angle ZOY form a linear pair. Find the value of x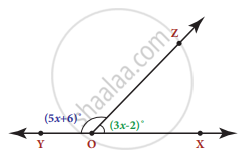
Draw a graph for the following data:
| Side of a square (cm) | 2 | 3 | 4 | 5 | 6 |
| Area (cm2) | 4 | 9 | 16 | 25 | 36 |
Does the graph represent a linear relation?
Challenging Problems
Three consecutive integers, when taken in increasing order and multiplied by 2, 3 and 4 respectively, total up to 74. Find the three numbers.
331 students went on a field trip. Six buses were filled to capacity and 7 students had to travel in a van. How many students were there in each bus?
A mobile vendor has 22 items, some which are pencils and others are ball pens. On a particular day, he is able to sell the pencils and ball pens. Pencils are sold for ₹ 15 each and ball pens are sold at ₹ 20 each. If the total sale amount with the vendor is ₹ 380, how many pencils did he sell?
Draw the graph of the lines y = x, y = 2x, y = 3x and y = 5x on the same graph sheet. Is there anything special that you find in these graphs?
Consider the number of angles of a convex polygon and the number of sides of that polygon. Tabulate as follows:
| Name of Polygon | No. of angles | No. of sides |
Use this to draw a graph illustrating the relationship between the number of angles and the number of sides of a polygon
Solutions for 3: Algebra
![Samacheer Kalvi solutions for Mathematics [English] Class 8 TN Board chapter 3 - Algebra Samacheer Kalvi solutions for Mathematics [English] Class 8 TN Board chapter 3 - Algebra - Shaalaa.com](/images/mathematics-english-class-8-tn-board_6:5f2b1b2038084cf381bfa42c826a928c.jpg)
Samacheer Kalvi solutions for Mathematics [English] Class 8 TN Board chapter 3 - Algebra
Shaalaa.com has the Tamil Nadu Board of Secondary Education Mathematics Mathematics [English] Class 8 TN Board Tamil Nadu Board of Secondary Education solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. Samacheer Kalvi solutions for Mathematics Mathematics [English] Class 8 TN Board Tamil Nadu Board of Secondary Education 3 (Algebra) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. Samacheer Kalvi textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Mathematics [English] Class 8 TN Board chapter 3 Algebra are Linear Equation in One Variable, Algebraic Expressions, Terms, Factors and Coefficients of Expression, Like and Unlike Terms, Types of Algebraic Expressions as Monomials, Binomials, Trinomials, and Polynomials, Multiplication of Algebraic Expressions, Multiplying Monomial by Monomials, Multiplying a Monomial by a Binomial, Multiplying a Monomial by a Trinomial, Multiplying a Binomial by a Binomial, Multiplying a Binomial by a Trinomial, Division of Algebraic Expressions, Dividing a Monomial by a Monomial, Dividing a Polynomial by a Monomial, Concept of Identity, Expansion of (a + b)2 = a2 + 2ab + b2, Expansion of (a - b)2 = a2 - 2ab + b2, Expansion of (a + b)(a - b) = a2-b2, Expansion of (x + a)(x + b), Expansion of (a + b)3, Expansion of (a - b)3, Expansion of (x + a)(x + b)(x + c), Factorising Algebraic Expressions, Factorisation by Taking Out Common Factors, Factorisation by Taking Out the Common Binomial Factor from Each Term, Factorisation by Regrouping Terms, Factorisation Using Identities, Factors of the Form (x + a)(x + b), Factorise Using the Identity (a + b)3, Factorise Using the Identity (a – b)3, Expressions with Variables, Concept of Equation, The Solution of an Equation, Graph, Cartesian Coordinate System, Co-ordinates of Points, Quadrants and Sign Convention, Plotting a Point in the Plane If Its Coordinates Are Given., Geometrical Representation of a Linear Equation by Plotting a Straight Line, Geometrical Representation of a Linear Equation by Line Parallel to the Coordinate Axes, Linear Pattern, Graph of a Linear Equation in Two Variables, Addition of Algebraic Expressions, Subtraction of Algebraic Expressions, Concept of Find the Error.
Using Samacheer Kalvi Mathematics [English] Class 8 TN Board solutions Algebra exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in Samacheer Kalvi Solutions are essential questions that can be asked in the final exam. Maximum Tamil Nadu Board of Secondary Education Mathematics [English] Class 8 TN Board students prefer Samacheer Kalvi Textbook Solutions to score more in exams.
Get the free view of Chapter 3, Algebra Mathematics [English] Class 8 TN Board additional questions for Mathematics Mathematics [English] Class 8 TN Board Tamil Nadu Board of Secondary Education, and you can use Shaalaa.com to keep it handy for your exam preparation.
