Advertisements
Advertisements
Question
The denominator of a fraction exceeds Its numerator by 8. If the numerator is increased by 17 and the denominator is decreased by 1, we get `3/2`. Find the original fraction.
Solution
Let the numerator & denominator be ‘n’ & ‘d’
Given that denominator exceeds numerator by 8
∴ d = n + 8 ...(1)
If numerator increased by 17 & denominator decreased by 1,
it becomes (n + 17) & (d – 1), fraction is `3/2`
i.e `("n" + 17)/("d" - 1) = 3/2` by cross multiplying, we get
`("n" + 17)/("d" - 1) = 3/2`
2(n + 17) = 3(d – 1)
2n + 2 × 17 = 3d – 3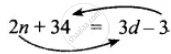
∴ 34 + 3 = 3d – 2n
∴ 3d – 2n = 37 ...(2)
Substituting equation (1) in (2), we get,
3 × (n + 8) – 2n = 37
3n + 3 × 8 – 2n = 37![]()
∴ n = 37 – 24 = 13
d = n + 8 = 13 + 8 = 21
The fraction is `"n"/"d" = 13/21`
APPEARS IN
RELATED QUESTIONS
Divide the given polynomial by the given monomial.
(3y8 − 4y6 + 5y4) ÷ y4
Write the degree of each of the following polynomials.
Write each of the following polynomials in the standard form. Also, write their degree.
(x3 − 1)(x3 − 4)
Divide 9x4 − 4x2 + 4 by 3x2 − 4x + 2 and find the quotient and remainder.
Verify the division algorithm i.e. Dividend = Divisor × Quotient + Remainder, in each of the following. Also, write the quotient and remainder.
| Dividend | Divisor |
| 14x2 + 13x − 15 | 7x − 4 |
Using division of polynomials, state whether
4x − 1 is a factor of 4x2 − 13x − 12
Using division of polynomials, state whether
z2 + 3 is a factor of z5 − 9z
Divide the first polynomial by the second in each of the following. Also, write the quotient and remainder:
3x2 + 4x + 5, x − 2
Find whether the first polynomial is a factor of the second.
4 − z, 3z2 − 13z + 4
Divide:
