Advertisements
Advertisements
Question
The sum of three numbers is 58. The second number is three times of two-fifth of the first number and the third number is 6 less than the first number. Find the three numbers
Solution
Here what we know
a + b + c = 58 ...(sum of three numbers is 58)
Let the first number be ‘x’
b = a × 3 ...(the second number is three times of the first `2/5` number)
b = `3 xx 2/5x = 6/5x`
Third number = x – 6
Sum of the numbers is given as 58.
∴ `x + 6/5x + (x - 6)` = 58
Multiplying by 5 throughout, we ge
5 × x + 6x + 5 × (x – 6) = 58 × 5
5x + 6x + 5x – 30 = 290
∴ 16x = 290 + 30
∴ 16x = 320
∴ x = `320/16`
x = 20
1st number = 20
2nd number = `3 xx 2/5 xx 20 = 6/5 xx 20` = 24
3rd number = 24 – 16 = 14
APPEARS IN
RELATED QUESTIONS
Find x: (7x – 5) – 4(2 + 5x) = 10(2 – x)
If a train runs at 60 km/hr it reaches its destination late by 15 minutes. But, if it runs at 85 kmph it is late by only 4 minutes. Find the distance to be covered by the train.
A mobile vendor has 22 items, some which are pencils and others are ball pens. On a particular day, he is able to sell the pencils and ball pens. Pencils are sold for ₹ 15 each and ball pens are sold at ₹ 20 each. If the total sale amount with the vendor is ₹ 380, how many pencils did he sell?
A piece of wire is ‘12s’ cm long. What will be the length of the side, if it is formed as an equilateral triangle
The rectangles made of identical square blocks with varying lengths but having only two square blocks as width are given below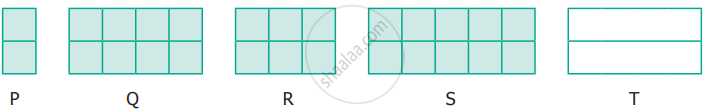
(i) How many small size squares are there in each of the rectangles P, Q, R and S?
(ii) Fill in the boxes
| Rectangle | P | Q | R | S | T |
| Number of small size squares along the breadth | 2 | 2 | ? | 2 | 2 |
| Number of squares along the length | 1 | 4 | 3 | ? | x |
| Total number of squares in rectangle | ? | 8 | ? | 10 | ? |
The product of ‘q’ and 20 is '20q'
The solution of the equation ax + b = 0 is ______.
x – 4 = – 2 has a solution ______.
In the equation 7k – 7 = 7, the variable is 7.
Translate the following statements into an equation:
The diameter (d) of a circle is twice its radius (r).
