Advertisements
Advertisements
Question
Write the negation of the following statement.
∀ n ∈ N, n + 3 > 9.
Solution
∃ n ∈ N such that n + 3 ≤ 9.
APPEARS IN
RELATED QUESTIONS
Using truth table prove that p ↔ q = (p ∧ q) ∨ (~p ∧ ~q).
Write the following compound statement symbolically.
Nagpur is in Maharashtra and Chennai is in Tamil Nadu.
Write the following compound statement symbolically.
Hima Das wins gold medal if and only if she runs fast.
Construct the truth table of the following statement pattern.
(p ∧ ∼q) ↔ (p → q)
Construct the truth table of the following statement pattern.
(p ∨ ∼ q) → (r ∧ p)
Construct the truth table of the following:
[(∼p ∨ q) ∧ (q → r)] → (p → r)
Express the following statement in symbolic form.
Milk is white or grass is green.
Write the negation of the following statement.
2 + 3 ≠ 5
Write the following statement in symbolic form.
It is not true that “i” is a real number.
Write the following statement in symbolic form.
Even though it is not cloudy, it is still raining.
Write the following statement in symbolic form.
Stock prices are high if and only if stocks are rising.
Write the following statement in symbolic form.
If Kutub-Minar is in Delhi then Taj-Mahal is in Agra.
Find the truth value of the following statement.
Every accountant is free to apply his own accounting rules if and only if machinery is an asset.
If p and q are true and r and s are false, find the truth value of the following compound statement.
p ∧ (q ∧ r)
Fill in the blanks :
Conjunction of two statement p and q is symbolically written as ______.
Assuming the first statement p and second as q. Write the following statement in symbolic form.
If Kiran drives the car, then Sameer will walk.
Assuming the first statement p and second as q. Write the following statement in symbolic form.
x3 + y3 = (x + y)3 if xy = 0.
If p : Proof is lengthy.
q : It is interesting.
Express the following statement in symbolic form.
Proof is lengthy and it is not interesting.
If p : Proof is lengthy.
q : It is interesting.
Express the following statement in symbolic form.
If proof is lengthy then it is interesting.
Let p : Sachin wins the match.
q : Sachin is a member of Rajya Sabha.
r : Sachin is happy.
Write the verbal statement of the following.
(p ∧ q) ∨ r
Let p : Sachin wins the match.
q : Sachin is a member of Rajya Sabha.
r : Sachin is happy.
Write the verbal statement of the following.
(p ∧ q) ∧ ∼ r
Let p : Sachin wins the match.
q : Sachin is a member of Rajya Sabha.
r : Sachin is happy.
Write the verbal statement of the following.
∼ (p ∨ q) ∧ r
Write the negation of the following.
Ramesh is intelligent and he is hard working.
Rewrite the following statement without using the connective ‘If ... then’.
If a quadrilateral is rhombus then it is not a square.
If p → q is an implication, then the implication ∼ q → ∼ p is called its
Find the negation of 10 + 20 = 30
Write the following statements in symbolic form
Even though it is not cloudy, it is still raining
Using truth table prove that p ˅ (q ˄ r) ≡ (p ˅ q) ˄ (p ˅ r)
Choose the correct alternative:
Negation of p → (p ˅ ~q) is
Write the following statements in symbolic form.
If Qutub – Minar is in Delhi then Taj-Mahal is in Agra
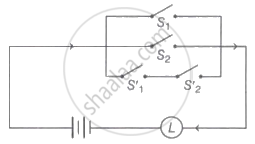
The symbolic form of the following circuit is (where p, q represents switches S1 and S2 closed respectively)
Let p : 7 is not greater than 4 and q : Paris is in France by two statements. Then ∼(p ∨ q) is the statement ______
The Boolean expression ∼(q ⇒ ∼p) is equivalent to: ______
Converse of the statement q `rightarrow` p is ______.
Write the following statement in symbolic form.
4 is an odd number if 3 is not a prime factor of 6.
If p, q are true statements and r, s are false statements, then write the truth value of the compound statement
(p `→` ∼ r) `→` (q ∧ s)
Using the statements
p: Seema is fat,
q: Seema is happy,
Write the following statements in symbolic form;
- Seema is thin and happy.
- If Seema is fat then she is unhappy.
Using truth table prove that:
~ (p `leftrightarrow` q) ≡ (p ∧ ~ q) ∨ (q ∧ ~ p)
