Angle Between Two Lines

Let `L_1` and `L_2` be two lines passing through the origin and with direction ratios `a_1, b_1, c_1` and `a_2, b_2, c_2`, respectively. Let P be a point on `L_1` and Q be a point on `L_2`. Consider the directed lines OP and OQ as given in following fig.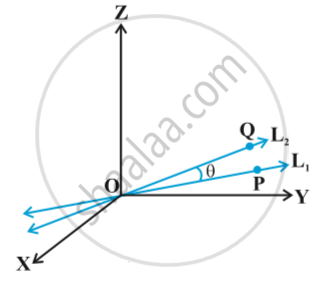 

Let θ be the acute angle between OP and OQ. Now recall that the directed line segments OP and OQ are vectors with components `a_1, b_1, c_1` and `a_2, b_2, c_2`, respectively. Therefore, the angle θ between them is given by
` cos θ = |(a_1a_2 + b_1b_2 + c_1c_2)/(sqrt(a_1^2 + b_1^2 + c_1^2) sqrt (a_2^2 + b_2^2 + c_2^2))| `    ...(1)
The angle between the lines in terms of sin θ is given by
`sin θ = sqrt (1- cos^2 θ) `

`= sqrt (1- (a_1a_2 + b_1b_2 + c_1c_2)^2 / ((a_1^2 + b_1^2 + c_1^2) (a_2^2 + b_2^2 + c_2^2)))`

`= sqrt ((a_1^2 + b_1^2 + c_1^2) (a_2^2 + b_2^2 + c_2^2) - (a_1a_2 + b_1b_2 + c_1c_2)^2 ) / (sqrt(a_1^2 + b_1^2 + c_1^2 )  sqrt(a_2^2 + b_2^2 +c_2^2))`

`= sqrt ((a_1 b_2 - a_2 b_1) ^2 + (b_1 c_2 - b_2 c_1)^ 2 + (c_1 a_2 - c_2 a_1) ^2 )/ (sqrt (a_1^2 + b_1^2 + c_1^2) sqrt (a_2^2 + b_2^2 + c_2^2))`  ...(2)`

If instead of direction ratios for the lines `L_1` and `L_2`, direction cosines, namely, `l_1, m_1, n_1` for `L_1` and `l_2, m_2, n_2` for `L_2` are given, then (1) and (2) takes the following form:
cos θ = `|l_1 l_2 + m_1m_2 + n_1n_2|`  `("as"  l_1^2 + m_1^2 + n_1^2 = 1 = l_2^2 + m_2^2 + n_2^2)`  ...(3)
and sin θ = `sqrt( (l_1m_2 - l_2m_1)^2 - (m_1n_2 - m_2n_1)^2 + (n_1l_2 - n_2 l_1)^2)`   ..(4)

Two lines with direction ratios `a_1, b_1, c_1` and `a_2, b_2, c_2` are
 (i) perpendicular i.e. if θ = 90° by (1) 
`a_1a_2 + b_1b_2 + c_1c_2 `= 0 
(ii) parallel i.e. if θ = 0 by (2)
`a_1/a_2 = b_1/b_2 = c_1/c_2`
Now, we find the angle between two lines when their equations are given. If θ is acute the angle between the lines
`vec r = vec a_1 + lambda vec b_1` and `vec r = vec a_2 + mu vec b _2`
then `cos theta = |(vec b _1 . vec b_2)/(|vec b _1|| vec b _2|)|`