Topics
Rational Numbers
- Rational Numbers
- Closure Property of Rational Numbers
- Commutative Property of Rational Numbers
- Associative Property of Rational Numbers
- Distributive Property of Multiplication Over Addition for Rational Numbers
- Identity of Addition and Multiplication of Rational Numbers
- Negative Or Additive Inverse of Rational Numbers
- Concept of Reciprocal or Multiplicative Inverse
- Rational Numbers on a Number Line
- Rational Numbers Between Two Rational Numbers
Linear Equations in One Variable
- Variable of Equation
- Concept of Equation
- Expressions with Variables
- Balancing an Equation
- The Solution of an Equation
- Linear Equation in One Variable
- Solving Equations Which Have Linear Expressions on One Side and Numbers on the Other Side
- Some Applications Solving Equations Which Have Linear Expressions on One Side and Numbers on the Other Side
- Solving Equations Having the Variable on Both Sides
- Some More Applications on the Basis of Solving Equations Having the Variable on Both Sides
- Reducing Equations to Simpler Form
- Equations Reducible to the Linear Form
Understanding Quadrilaterals
- Concept of Curves
- Different Types of Curves - Closed Curve, Open Curve, Simple Curve.
- Concept of Polygons
- Classification of Polygons
- Properties of a Quadrilateral
- Interior Angles of a Polygon
- Exterior Angles of a Polygon and Its Property
- Concept of Quadrilaterals
- Properties of Trapezium
- Properties of Kite
- Properties of a Parallelogram
- Properties of Rhombus
- Property: The Opposite Sides of a Parallelogram Are of Equal Length.
- Property: The Opposite Angles of a Parallelogram Are of Equal Measure.
- Property: The adjacent angles in a parallelogram are supplementary.
- Property: The diagonals of a parallelogram bisect each other. (at the point of their intersection)
- Property: The diagonals of a rhombus are perpendicular bisectors of one another.
- Property: The Diagonals of a Rectangle Are of Equal Length.
- Properties of Rectangle
- Properties of a Square
- Property: The diagonals of a square are perpendicular bisectors of each other.
Practical Geometry
- Introduction to Geometric Tool
- Constructing a Quadrilateral When the Lengths of Four Sides and a Diagonal Are Given
- Constructing a Quadrilateral When Two Diagonals and Three Sides Are Given
- Constructing a Quadrilateral When Two Adjacent Sides and Three Angles Are Known
- Constructing a Quadrilateral When Three Sides and Two Included Angles Are Given
- Some Special Cases
Data Handling
- Concept of Data Handling
- Interpretation of a Pictograph
- Interpretation of Bar Graphs
- Drawing a Bar Graph
- Interpretation of a Double Bar Graph
- Drawing a Double Bar Graph
- Organisation of Data
- Frequency Distribution Table
- Graphical Representation of Data as Histograms
- Concept of Pie Graph (Or a Circle-graph)
- Interpretation of Pie Diagram
- Chance and Probability - Chance
- Basic Ideas of Probability
Squares and Square Roots
- Concept of Square Number
- Properties of Square Numbers
- Some More Interesting Patterns of Square Number
- Finding the Square of a Number
- Concept of Square Roots
- Finding Square Root Through Repeated Subtraction
- Finding Square Root Through Prime Factorisation
- Finding Square Root by Division Method
- Square Root of Decimal Numbers
- Estimating Square Root
Cubes and Cube Roots
Comparing Quantities
- Concept of Ratio
- Basic Concept of Percentage
- Increase Or Decrease as Percent
- Concept of Discount
- Estimation in Percentages
- Basic Concepts of Profit and Loss
- Sales Tax, Value Added Tax, and Good and Services Tax
- Calculation of Interest
- Concept of Compound Interest
- Deducing a Formula for Compound Interest
- Rate Compounded Annually Or Half Yearly (Semi Annually)
- Applications of Compound Interest Formula
Algebraic Expressions and Identities
- Algebraic Expressions
- Terms, Factors and Coefficients of Expression
- Types of Algebraic Expressions as Monomials, Binomials, Trinomials, and Polynomials
- Like and Unlike Terms
- Addition of Algebraic Expressions
- Subtraction of Algebraic Expressions
- Multiplication of Algebraic Expressions
- Multiplying Monomial by Monomials
- Multiplying a Monomial by a Binomial
- Multiplying a Monomial by a Trinomial
- Multiplying a Binomial by a Binomial
- Multiplying a Binomial by a Trinomial
- Concept of Identity
- Expansion of (a + b)2 = a2 + 2ab + b2
- Expansion of (a - b)2 = a2 - 2ab + b2
- Expansion of (a + b)(a - b) = a2-b2
- Expansion of (x + a)(x + b)
Mensuration
Visualizing Solid Shapes
Exponents and Powers
Direct and Inverse Proportions
Factorization
- Factors and Multiples
- Factorising Algebraic Expressions
- Factorisation by Taking Out Common Factors
- Factorisation by Regrouping Terms
- Factorisation Using Identities
- Factors of the Form (x + a)(x + b)
- Dividing a Monomial by a Monomial
- Dividing a Polynomial by a Monomial
- Dividing a Polynomial by a Polynomial
- Concept of Find the Error
Introduction to Graphs
- Concept of Bar Graph
- Interpretation of Bar Graphs
- Drawing a Bar Graph
- Concept of Double Bar Graph
- Interpretation of a Double Bar Graph
- Drawing a Double Bar Graph
- Concept of Pie Graph (Or a Circle-graph)
- Graphical Representation of Data as Histograms
- Concept of a Line Graph
- Linear Graphs
- Some Application of Linear Graphs
Playing with Numbers
Notes
Some Interesting Patterns of Cube Numbers:
1. Adding consecutive odd numbers:
1 = 1 = 13
3 + 5 = 8 = 23
7 + 9 + 11 = 27 = 33
13 + 15 + 17 + 19 = 64 = 43
21 + 23 + 25 + 27 + 29 = 125 = 53
We can see from the above pattern,
If we want n3, then we needed n consecutive odd numbers such that the sum of consecutive odd numbers is equal to n3.
2. Cubes and their prime factors:
Consider the following prime factorisation of the numbers and their cubes.
| Prime factorisation of a number | Prime factorisation of its cube |
| 4 = 2 × 2 | 43 = 64 = 2 × 2 × 2 × 2 × 2 × 2 = 23 × 23. |
| 6 = 2 × 3 | 63 = 216 = 2 × 2 × 2 × 3 × 3 × 3 = 23 × 33. |
| 15 = 3 × 5 | 153 = 3375 = 3 × 3 × 3 × 5 × 5 × 5 = 33 × 53. |
| 12 = 2 × 2 × 3 | 123 = 1728 = 2 × 2 × 2 × 2 × 2 × 2 × 3 × 3 × 3 = 23 × 23 × 33. |
Observe that each prime factor of a number appears three times in the prime factorisation of its cube.
If in the prime factorisation of any number each factor appears three times, then the number is a perfect cube.
1) Is 216 a perfect cube?
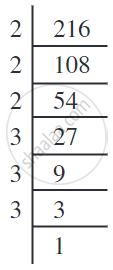
By prime factorisation, 216 = 2 × 2 × 2 × 3 × 3 × 3....(factors can be grouped in triples)
Each factor appears 3 times.
216 = 23 × 33 = (2 × 3)3 = 63 which is a perfect cube.
3) Smallest multiple that is a perfect cube
1) Is 392 a perfect cube? If not, find the smallest natural number by which 392 must be multiplied so that the product is a perfect cube.
Solution:
392 = 2 × 2 × 2 × 7 × 7
The prime factor 7 does not appear in a group of three. Therefore, 392 is not a perfect cube. To make its a cube, we need one more 7. In that case
392 × 7 = 2 × 2 × 2 × 7 × 7 × 7 = 2744 which is a perfect cube.
Hence the smallest natural number by which 392 should be multiplied to make a perfect cube is 7.
Example
Is 243 a perfect cube?
243 = 3 × 3 × 3 × 3 × 3
In the above factorisation 3 × 3 remains after grouping the 3’s in triplets.
Therefore, 243 is not a perfect cube.
Example
Is 53240 a perfect cube? If not, then by which smallest natural number should 53240 be divided so that the quotient is a perfect cube?
53240 = 2 × 2 × 2 × 11 × 11 × 11 × 5
The prime factor 5 does not appear in a group of three. So, 53240 is not a perfect cube. In the factorisation 5 appears only one time. If we divide the number by 5, then the prime factorisation of the quotient will not contain 5.
So, 53240 ÷ 5 = 2 × 2 × 2 × 11 × 11 × 11
Hence the smallest number by which 53240 should be divided to make it a perfect
cube is 5.
The perfect cube, in that case, is = 10648.
Example
Is 1188 a perfect cube? If not, by which smallest natural number should 1188 be divided so that the quotient is a perfect cube?
1188 = 2 × 2 × 3 × 3 × 3 × 11
The primes 2 and 11 do not appear in groups of three. So, 1188 is not a perfect cube. In the factorisation of 1188, prime 2 appears only two times and the prime 11 appears once. So, if we divide 1188 by 2 × 2 × 11 = 44, then the prime factorisation of the quotient will not contain 2 and 11.
Hence the smallest natural number by which 1188 should be divided to make it a
a perfect cube is 44.
And the resulting perfect cube is 1188 ÷ 44 = 27 (= 33).
Example
Is 68600 a perfect cube? If not, find the smallest number by which 68600 must be multiplied to get a perfect cube.
We have, 68600 = 2 × 2 × 2 × 5 × 5 × 7 × 7 × 7.
In this factorisation, we find that there is no triplet of 5.
So, 68600 is not a perfect cube.
To make it a perfect cube we multiply it by 5.
Thus,
68600 × 5 = 2 × 2 × 2 × 5 × 5 × 5 × 7 × 7 × 7
= 343000, which is a perfect cube.
If you would like to contribute notes or other learning material, please submit them using the button below.
Shaalaa.com | Patterns of Cube Numbers - Part 1
to track your progress
Series: Some Interesting Patterns of Cube Numbers
0%
