Advertisements
Advertisements
प्रश्न
A (2, 5), B (–1, 2) and C (5, 8) are the co-ordinates of the vertices of the triangle ABC. Points P and Q lie on AB and AC respectively, such that : AP : PB = AQ : QC = 1 : 2.
- Calculate the co-ordinates of P and Q.
- Show that : `PQ = 1/3 BC`.
उत्तर
i. Co-ordinates of P are
`((1 xx (-1) + 2 xx 2)/(1 + 2),(1 xx 2 + 2 xx 5)/(1 xx 2))`
= `(3/3, 12/3)`
= (1, 4)
Co-ordinates of Q are
`((1 xx 5 + 2 xx 2)/(1 + 2),(1 xx 8 + 2 xx 5)/(1 + 2))`
= `(9/3, 18/3)`
= (3, 6)
ii. Using distance formula, we have:
`BC = sqrt((5 + 1)^2 + (8 - 2)^2)`
`BC = sqrt(36 + 36)`
`BC = 6sqrt(2)`
`PQ = sqrt((3 - 1)^2 + (6 - 4)^2)`
`PQ = sqrt(4 + 4)`
`PQ = 2sqrt(2)`
Hence, `PQ = 1/3 BC`.
APPEARS IN
संबंधित प्रश्न
If the point C (–1, 2) divides internally the line segment joining A (2, 5) and B in ratio 3 : 4, find the coordinates of B
If the points A (6, 1), B (8, 2), C(9, 4) and D(p, 3) are vertices of a parallelogram, taken in order, find the value of p
If (1, 2), (4, y), (x, 6) and (3, 5) are the vertices of a parallelogram taken in order, find x and y.
If the points (-2, -1), (1, 0), (x, 3) and (1, y) form a parallelogram, find the values of x and y.
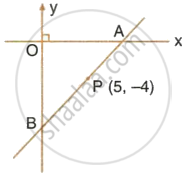
The point P (5, – 4) divides the line segment AB, as shown in the figure, in the ratio 2 : 5. Find the co-ordinates of points A and B. Given AP is smaller than BP.
In Figure 2, P (5, −3) and Q (3, y) are the points of trisection of the line segment joining A (7, −2) and B (1, −5). Then y equals

Find the coordinate of a point P which divides the line segment joining :
D(-7, 9) and E( 15, -2) in the ratio 4:7.
The vertices of a parallelogram in order are A(1, 2), B(4, y), C(x, 6) and D(3, 5). Then (x, y) is ______.
In what ratio does the x-axis divide the line segment joining the points (– 4, – 6) and (–1, 7)? Find the coordinates of the point of division.
If (2, 4) is the mid-point of the line segment joining (6, 3) and (a, 5), then the value of a is ______.
